The text that follows is a PREPRINT.
Please cite
as:
Fearnside, P.M.
1997. Greenhouse-gas emissions from
Amazonian hydroelectric reservoirs: The example of
ISSN: 0376-8929
Copyright:
Cambridge University Press
The original publication is available at http://journals.cambridge.org/ <publisher link>
GREENHOUSE-GAS EMISSIONS FROM AMAZONIAN HYDROELECTRIC
RESERVOIRS: THE EXAMPLE OF BRAZIL'S TUCURUÍ DAM AS COMPARED TO FOSSIL FUEL
ALTERNATIVES
Philip M. Fearnside
National Institute for Research
in the Amazon (INPA)
C.P. 478
69011-970 Manaus, Amazonas
BRAZIL
Fax: 55 - 92 – 642-8909
E-mail:
pmfearn@inpa.gov.br
28 Mar. 1997
Text published in Environmental Conservation 24(1): 64-75. (1997)
Running head: Tucuruí Greenhouse-Gas Emissions
Correspondence: Dr. Philip M. Fearnside, Fax: +55 92 642-8909,
E-mail: pmfearn@inpa.gov.br
SUMMARY
Hydroelectric dams in tropical forest areas emit carbon dioxide and methane. How these emissions and their impacts should be calculated, and how comparisons should be made with global warming contributions of alternative energy sources such as fossil fuels, can lead to sharp differences in conclusions on the relative advantages of these options. The example of Brazil's Tucuruí Dam is examined to clarify these differences. The present paper extends an earlier analysis to 100 years and explores the differences between these and comparable fossil fuel emissions.
Factors considered here in calculating emissions for Tucuruí Dam include the initial stock and distribution of carbon, decay rates and pathways (leading to carbon dioxide and methane), and losses of power in transmission lines. Factors not considered include forest degradation on islands and reservoir shores, nitrous oxide sources in drawdown zones and transmission lines, additional methane emission pathways for release from standing trees, water passing through the turbines, etc. Construction-phase emissions are also not included; neither are emissions from deforestation by people displaced by and attracted to the project. A complete accounting of the alternative landscape is also lacking. Standardization of the level of reliability of the electricity supply is needed to compare hydroelectric and thermoelectric options.
Types of emissions calculations commonly used include the ultimate contribution to emissions, the annual balance of emissions in a given year, and emissions over a long time horizon (such as 100 years). The timing of emissions differs between hydroelectric and thermal generation, hydro producing a large pulse of carbon dioxide emissions in the first years after filling the reservoir while thermal produces a constant flux of gases in proportion to the power generated. The impacts of emissions are related to the atmospheric load (stocks) of the gases rather than to the emissions (flows), and therefore last over a long time. According to the calculations in the present paper, the average carbon dioxide molecule in the atmospheric load contributed by Tucuruí was present in the atmosphere 15 years earlier than the average molecule in the comparable load from fossil fuel generation. This means that, considering a 100-year time horizon, a tonne of CO2 emitted by Tucuruí has 15% more global warming impact than a tonne emitted by fossil fuel, assuming no discounting. If discounting is applied, then the relative impact of the hydroelectric option is increased.
Time preference, either by discounting or by an alternative procedure, is a key factor affecting the attractiveness of hydroelectric power. At low annual discount rates (say 1-2%) the attractiveness of Tucuruí, although less than without discounting, is still 3-4 times better than fossil fuel generation. If the discount rate reaches 15%, the situation is reversed, and fossil fuel generation becomes more attractive from a global warming perspective. Tucuruí, with a power density (installed capacity/reservoir area) of 1.63 W m-2 is better than both the 0.81 W m-2 average for Brazilian Amazonia's 5500 km2 of existing reservoirs and the 1 W m-2 estimated by Brazil's electrical authorities as the mean for all planned hydroelectric development in the region.
KEYWORDS: greenhouse gases; hydroelectric dams; global warming; reservoirs; carbon emissions; rainforest
INTRODUCTION
Hydroelectric dams in tropical forest areas emit greenhouse gases such as carbon dioxide (CO2) and methane (CH4). How these emissions and their impacts should be calculated, and how comparisons should be made with global warming contributions of other energy sources such as fossil fuels, is a matter of disagreement.
The proportion of the carbon in the decomposing biomass that is emitted as CH4 rather than CO2 strongly influences the global warming impact of reservoirs. Per tonne of carbon, CH4 is much more potent than CO2 in provoking the greenhouse effect. The average lifetime of CH4 in the atmosphere is much shorter than that of CO2, i.e. 14.5 years versus 125 years, given an atmosphere of constant composition as assumed by the Intergovernmental Panel on Climate Change (IPCC) (Albritton et al. 1995, p. 222).
The present paper examines the example of Brazil's Tucuruí Dam in order to clarify differences among various approaches to this problem. The analysis builds on a previous one (Fearnside 1995) that calculated emission of this and other Amazonian hydroelectric dams for a single year, namely 1990, which is the base year for emissions inventories now in progress under the Framework Convention on Climate Change (FCCC). The present paper is limited to the case of Tucuruí, but I stress that the intent is not to portray this dam as typical of either existing or planned Amazonian dams. Tucuruí is better, from a greenhouse-gas perspective, than either the average existing dam or the average planned dam, but it does not represent an extreme case. Considering official values for reservoir areas, Tucuruí has 1.63 watts (W) of installed capacity per m2 of reservoir surface, whereas ELETROBRÁS (Brazil's national electrical authority) considers the average power density for the entire hydroelectric potential of the Amazon Region to be only one W m-2 (Rosa et al. 1996a, p. 6). The equivalent figure for the 5537 km2 of water surface in the four existing large dams (whose total installed capacity is 4490 MW) is 0.81 W m-2, or only half the power density of Tucuruí.
The 2247 km2 Tucuruí Dam was closed in 1984 on the Tocantins River, and became the first major hydroelectric project in Brazilian Amazonia (Fig. 1). Only the 72 km2 Curuá-Una Dam, closed in 1977, had preceded it in the region. Subsequently dams were closed in 1987 at Balbina (3147 km2) and in 1988 at Samuel (465 km2) (areas from LANDSAT-TM imagery, see Fearnside 1995). Planned reservoirs listed in Brazil's 2010 Plan, irrespective of the expected date of construction, constitute a total area of 100 000 km2 (ELETROBRÁS 1987, p. 150), which is c. 20 times the present total of 5931 km2. The above areas of existing reservoirs are those measured from LANDSAT imagery, and differ slightly from 'official' values (Fearnside 1995, p. 11).
[Figure 1 here]
Types of emissions calculations
Ultimate contribution to emissions. One way to approach greenhouse gas (GHG) emissions from hydroelectric dams is to calculate the ultimate contribution that would be obtained with decomposition of all forest biomass flooded by the reservoir. This is much easier to calculate than is the impact of flooding on the annual balance of net emissions because we need not know the rate at which decomposition occurs. Rosa & Schaeffer (1995) have done a calculation for Tucuruí using a method equivalent to this approach, assuming that biomass has a half-life of only seven years and considering emissions over a 100-year time horizon without discounting. The assumptions of these authors can be used to calculate that the cumulative release over 100 years would be 2.3-5.3 million t of CH4, or 56.4-128.9 million t of CO2-equivalent gas using 1994 IPPC 100-year integration global warming potentials (GWPs). Rosa & Schaeffer's (1995) analysis, however, assumes (without explanation of any justification) that 10-30% of the biomass decomposes anaerobically (i.e. to CH4), and considers only the impact of CH4, thereby ignoring the 70-90% of the carbon that they have assumed is released as CO2. A valid comparison would require accounting for all gases emitted by both options (see Fearnside 1996b and Rosa et al. 1996b).
Calculation of the ultimate contribution of reservoirs to emissions, while useful as an illustration, tells us little about the contribution to the annual balance of emissions. The United Nations Framework Convention on Climate Change (FCCC), signed at the United Nations Conference on Environment and Development (UNCED) in Rio de Janeiro in June 1992 by 155 countries plus the European Union, stipulates that each nation must make an inventory of carbon stocks and fluxes of greenhouse gases. This implies that the annual balance of GHG fluxes will be the criterion adopted for assigning responsibility amongst nations for global warming. Because forest biomass in Amazonian reservoirs decays exceedingly slowly, the contribution to the annual balance is very different from the ultimate potential for emitting carbon.
Junk & de Mello (1987, p. 381) made two calculations of the emissions of Tucuruí as compared to fossil fuel generation. In an optimistic calculation, they concluded that the quantity of CO2 released from forest flooded in Tucuruí would be emitted by generating the same amount of energy from fossil fuels in only 1.5 y. This calculation assumed that mean biomass is 300 t ha-1, that no forest is cleared outside of the reservoir area, and that the dam would generate from the outset 8000 MW of power (i.e. the Tucuruí-II configuration and the [impossible] load factor of 100%).
The pessimistic calculation of Junk & de Mello (1987, p. 381) indicated breakeven after 37 years. The latter calculation was made assuming that mean biomass is 600 t ha-1, that an area outside the reservoir is cleared equal to five times the reservoir area, and that 4000 MW are generated from the outset. Both the optimistic and pessimistic calculations assumed that only half the area of the reservoir was forested, that all forest biomass carbon is emitted as CO2, and that the thermoelectric alternative burns diesel fuel with an energy content of 10 900 kcal kg-1 and a conversion efficiency of 20% to electric power.
Annual balance of emissions in a specific year. Under the Framework Convention on Climate Change (FCCC), countries are currently undertaking national emissions inventories to assess fluxes in the year 1990--an exercise to be repeated at regular intervals for future years. Ability to assess fluxes for a specific year, such as 1990, is therefore important. The approximate quantities of biomass and emissions present in each zone in Tucuruí were calculated for 1990 (the base year for national inventories--Fearnside 1995, Table VII); Tucuruí's methane emission in 1990 totalled 0.09 million t CH4 gas. Emissions of CH4 gas from the entire reservoir were 215 t from termites, 39 800 t from open water, 14 300 t from macrophyte beds, and 40 200 t from underwater decay. The underwater decay portion was composed of contributions from the permanently inundated zone of 0 t from wood in the surface water zone, 11 900 t from wood in the anoxic water zone, 2000 t from leaves and other non-wood biomass in the anoxic water zone, and 10 200 t from below-ground biomass. Contributions from the seasonally-inundated zone were 13 300 t from underwater decay of wood, 1 800 t from leaves and other non-wood biomass, and 64 t from below-ground decay.
Emissions over 100 years
Timing of emissions. Hydroelectric power has some fundamental differences from fossil fuels that make comparisons of impacts of these two options produce very different results depending on the treatment given to time in the calculation method. Fossil fuel generation produces emissions in direct proportion to energy produced, such that supplying a constant stream of benefits in the form of electricity will produce a constant stream of emissions. Hydroelectric dams in tropical forest areas, on the other hand, produce a large pulse of emissions in the first few years after closing, and emissions then taper off to a much lower level as the bulk of forest biomass, especially the above-water biomass, either decomposes or is transferred to the bottom of the reservoir. The benefits typically follow a pattern that is the inverse of the pattern for the impacts. Benefits begin at a low level with only a few turbines installed, and increase stepwise over the succeeding several years as the remaining turbines are installed.
METHODS
Factors included in calculating emissions for Tucuruí
Initial stock and distribution of carbon. Areas of different forest types and the biomass of each were estimated by the National Institute for Research in the Amazon (INPA) as part of the environmental studies contracted by ELETRONORTE (Revilla Cardenas et al. 1982, p. 90). The area-weighted average above-ground biomass is 394 t ha-1 (oven dry-weight), while the approximate total biomass is 517 t ha-1. Apportionment of the biomass into vertical zones was based on the depths of water at the normal and minimum operating levels (see Appendix).
Logging removals prior to flooding were small (Fearnside 1990). Of the above-ground biomass, 1% was assumed to have been removed (Fearnside 1995). Tucuruí has been known for the underwater logging activity initiated in 1988 using a specially developed underwater chainsaw. However, negotiations to use biomass from this source on a wide scale to supply charcoal to pig iron smelters in the Grande Carajás Program area have broken down. Nevertheless, the present calculation assumes that between 1988 and 2000, half of the biomass is removed.
Decay rates and pathways. The reservoir is divided into two horizontal zones, namely those permanently flooded and those seasonally flooded (Fearnside 1995, Fig. 3). The biomass is allocated into four vertical zones: aerial, surface water, anoxic water, and under-ground (following Fearnside 1995, Table V), using the vertical distribution of biomass in forest studied near Manaus by Klinge & Rodrigues (1973; see Fearnside 1995, Table IV). Based on the proportions of biomass in vertical strata, the water depths at minimum and maximum operating levels, and the areas in each zone, the biomass is calculated in five categories, namely above-water wood, surface water wood, anoxic water wood, anoxic water leaves and other non-wood, which are all assumed to fall to the bottom, and below-ground wood. The progression of biomass values is calculated for each year, zone and biomass component. This is done using rates of decay in each zone and rates of biomass falling from the above-water to the below-water zones. The parameters used for calculating emissions from Tucuruí are the same as those used in the previous analysis (Fearnside 1995, Table VI). I emphasize that a number of the parameters regarding underwater decay rates are based on assumptions, but that rates for the above-water decay that generates the bulk of emissions are based on measurements. These measurements are those available for decay in Amazonian clearings (Buschbacher 1984; C. Uhl & J. Saldariagga unpublished manuscript; see Fearnside 1996a).
The initial biomass present is estimated at 291.4 t ha-1 of wood in the above-water zone, 5.33 t ha-1 of wood in the surface water zone (to 1 m depth at the minimum water-level), 55.47 t ha-1 of wood and other non-wood components in the anoxic water zone, and 122.69 t ha-1 of below-ground wood. The biomass estimate is based on measurements made in the Tucuruí reservoir area prior to flooding (Revilla Cardenas et al. 1982) and the allocation into vertical zones is based on a study near Manaus (Klinge & Rodrigues 1973). The above-water and below-ground zones cover the entire forested part of the reservoir (1926 km2), while the surface water and anoxic water zones (using mean values for depth) cover the forested portion of the permanently-flooded zone (858 km2).
Methane emission from termites involved in the decomposition of wood projecting out of the water is also calculated, following Martius et al. (1996). This emission is limited by small size of the population of termites that is able to establish itself before the biomass is removed through other processes.
Methane is also produced from the 'water' in hydroelectric dams, representing that coming from dissolved carbon, soil organic matter, and decomposition of macrophytes and other organisms. Since measurements for such emissions are lacking, a value derived from studies in Amazonian floodplain (várzea) lakes is used instead (see Fearnside 1995, Table VIII). This is 53.9 mg CH4 m-2 day-1 for the open water, and 174.7 mg CH4 m-2 day-1 for macrophyte beds. Tucuruí is assumed to be 90% open water and 10% macrophyte beds. LANDSAT-based measurements by Novo and Tundisi (1994, p. 149) indicated that the Tucuruí reservoir was 67% open water, 22% emergent dead trees and macrophytes, and 11% seasonally-flooded area. A CH4 emission estimate for the reservoir was derived by multiplying these areas by values for emissions per unit of area from measurements made by Bartlett et al. (1990) in similar habitats in várzea lakes (Novo & Tundisi 1994). The CH4 flux rates used in the present study are slightly lower, based on the Bartlett et al. (1990) results averaged with other available measurements from várzea lakes (Fearnside 1995, p. 15).
Transmission losses. Transmission loss must be included in any calculation to have a fair comparison of hydroelectric energy with fossil fuel energy. Thermoelectric plants generate electricity at the site where it will be used, and losses from its local distribution networks can be assumed to be equal to those from local distribution of hydropower. The long-distance transport from the hydroelectric site to the point of consumption applies only to hydro, and must be considered. In the present calculation, a loss of 2.5% is used, this being the low value assumed in the viability study of the Balbina Dam (ELETRONORTE/MONASA/ENGE‑RIO 1976). Tucuruí has 743 km of 500-kilovolt (kV) transmission line and 75 km of 230-kV line, not counting the c. 500 km 500-kV line segment from Imperatriz to São Luís (ELETRONORTE no date [c.1983]). The total is thus over four times the length of Balbina's 190-km 230-kV line, but transmits much larger volumes of energy. Transmission losses were not included in the calculations for 1990 in Fearnside (1995).
RESULTS
Emissions
The emissions from the Tucuruí-I phase are shown in Fig. 2 over a span of 100 years, and a great pulse of CO2 emissions in the first years after filling the reservoir is evident. Methane, under current assumptions, is emitted at an almost constant rate over the time horizon. In Fig. 3, the effect of Tucuruí and its fossil fuel equivalent are compared in terms of global warming impact of the annual emissions, as expressed in terms of CO2-equivalents adjusted to the year of emission; these CO2 equivalents are therefore instantaneous values without adjustment for the effects of non-simultaneous emissions over the course of a time horizon of, say, 100 years. These CO2 equivalents are computed by multiplying the quantities of each gas by its 1994 IPCC 100-year integration GWP (Albritton et al. 1995, p. 222). On this kind of instantaneous basis, i.e. forgiving the accumulated history of emissions since the beginning of the time series, Tucuruí begins to 'break even' from the sixth year onwards. In Figs. 2 and 3, time 0 signifies the time the power station comes on line, not the time the dam is closed. Emissions from the filling phase (i.e. before the dam comes on line), plus emissions from construction, would be represented by negative numbers for time.
[Figures 2 and 3 here]
The atmospheric loads of GHGs from Tucuruí are shown in Fig. 4a over a time horizon of 100 years. The comparable profile for emissions from fossil fuel generation of the same power is shown in Fig. 4b. The effect of the great pulse of initial emissions in the case of hydroelectric generation is to maintain a higher level of CO2 in the atmosphere for a period after the dam begins to 'break even' on an instantaneous basis (Fig. 3).
[Figure 4 here]
The average radiatively-equivalent CO2 molecule emitted by a hydroelectric dam is present in the atmosphere earlier than the corresponding molecule emitted by fossil fuel generation. 'Radiatively equivalent CO2' refers to equivalents of CO2 in terms of instantaneous radiative forcing (not GWP, over a long time horizon, such as the IPCC 100-year integration GWPs). The 'center of gravity' of the distribution of total radiative forcing (Fig. 5) is year 52 for Tucuruí's hydroelectric output, and year 67 for the fossil-fuel equivalent of Tucuruí. The 15-year difference represents a significant gain in postponing global warming. The value attached to this time difference depends on the discount rate chosen (Fig. 5).
[Figure 5 here]
The effect of the discount rate on the relative advantage of hydroelectric generation at Tucuruí versus its thermoelectric equivalent is shown in Figure 6. The benefit/cost ratio declines to a value of one at an annual discount rate of 15%. Were the impacts for hydroelectric generation to include the emissions from dam construction and other sources not included in the present calculation, the curve would be shifted to the left and benefits would be equal to impacts at a lower value for discount rate.
[Figure 6 here]
DISCUSSION
Factors not considered in the present calculation
Forest degradation on islands and shores. Forest on islands and on the reservoir's shores are subjected to stress from the raised water table, causing many individual trees to die, and depleting the forest's biomass. Forest degradation on islands also occurs due to the effect of forest fragmenting into small isolated patches (e.g. Lovejoy et al. 1984).
Nitrous oxide sources. Nitrous oxide (N2O) is probably released from soils exposed in the seasonally-flooded zone during drawdown periods. Some N2O is also formed in the air by high-voltage transmission lines. Quantification of N2O is important because of the high impact of this gas on global warming relative to CO2; its 100-year integration global warming potential (GWP) is 320 relative to CO2 on a mass basis (Albritton et al. 1995, p. 222).
Additional methane emission pathways. The processes by which CH4 is released are not well quantified, and could significantly increase the amount of these emissions above what has been calculated in the present paper. Emissions here have been estimated based on different processes. For emissions from the water, this is on the basis of available information on emission from the water surface of várzea lakes. However, much of the CH4 is oxidized to CO2 in the water column before being released at the surface. Processes unique to reservoirs that would allow CH4 to be released directly, without passing through the full water column, would substantially increase emissions over this estimate. One such contribution is CH4 released when water passes through the turbines, taking anoxic water and abruptly decreasing its pressure. With an average streamflow of 11 100 m3 s-1, Tucuruí's volume of 48 ´ 109 m3 of water turns over every 50 days (0.138 years). Marc Lucotte (personal communication) found only a few percent of the total CH4 release to occur through water passed through the turbines of the 15000 km2 La Grande complex in Quebec, Canada. However, because La Grande has such a large area, and because its turnover time is c. one year, the relative importance of water surface would be greater than at Tucuruí. The reason is the same as that causing natural lakes, in general, to have greater contribution from wind and surface diffusion than do reservoirs (see Baxter 1977, p. 259).
The amount of CH4 emitted by the reservoir depends heavily on the available routes through which CH4 in anoxic water at the reservoir bottom can reach the surface without being oxidized to CO2 in the water column. The present calculation considers only diffusion through the water surface at a rate assumed to be equal to that occurring in várzea lakes. Individual events that bring anoxic water to the surface would not be captured by these relatively low mean rates. Cold spells (friagens) affect the western part of Brazilian Amazonia, but not the location of Tucuruí in eastern Amazonia. Cold spells cause breakage of the thermocline and complete mixing of the water column, bringing anoxic CH4-rich water to the surface where a pulse of emissions can occur. However, at Tucuruí the river channel portion of the reservoir has been found to be thermally stratified only in the dry season; with the onset of the rains, the great influx of oxygenated rain-water eliminates anoxic conditions in the channel during the high-water period when the turnover time is only a few weeks (Junk & de Mello 1987, p. 380). In Tucuruí's stagnant bays and littoral areas where standing trees impede water flow, stratification is maintained throughout the year (Pereira 1989 cited by Roulet 1992, p. 52). The same applies to the Brokopondo reservoir in Surinam (Leentvaar 1966 cited by Baxter 1977, p. 261).
The CH4 release calculations in the present paper do not include the possible role of dead trees standing in the reservoir in serving as conduits for CH4 from the soil of the reservoir floor. Marc Lucotte (personal communication 1996), has found dead trees in reservoirs in northern Canada to act in this way, with CH4 passing through the xylem and phloem of the dead trees allowing the gas to be released directly to the atmosphere, thereby escaping blockage by the thermocline and oxidation to CO2 in the water column.
Construction-phase emissions. Construction of hydroelectric dams emits greenhouse gases through fuel use in excavation of earth and rock, transport of materials, and emissions in the manufacture of cement and steel. Tucuruí required 6.2 million m3 of concrete (ELETRONORTE no date [1992], p. 5). In addition there were 55.3 million m3 of compacted clay, 20.0 million m3 of rockfill, 22.9 million m3 of rock excavation, 24.3 million m3 of ordinary excavation, and 4.7 million m3 of filters and transitions (ELETRONORTE no date [c.1983]). For the transmission line, 1937 towers were required, not counting the Imperatriz-São Luís stretch of the line.
Complete accounting of alternative landscape. One must have an estimate of the emissions that would have occurred in the absence of the dam. The easiest assumption in estimating such an emission is that the landscape remained in a static state equal to that present before building the dam, but a fairer comparison would be achieved by comparing the dam with a scenario for development in the region without the dam.
Primary forest soils are natural methane sinks, and removing this sink represents a small impact on global warming (Keller et al. 1986). This is included in calculations of contributions of hydroelectric dams (along with deforestation) to the annual balance of net emissions in 1990 (Fearnside 1996a), but is not included in the Tucuruí calculation in the present paper.
Possible uptake of carbon by growth of natural forest, found in the one available measurement so far (Grace et al. 1995), has not been included in the calculation. Were the forest considered to be a natural carbon sink, then removing it by flooding would have greater impact on global warming.
Land-use emissions from deforestation influenced by the dam can have a significant impact on the net effect of the dam. Because the human population that was displaced by the reservoir would have continued to clear within the submergence area had the dam not been built, only the initial pulse of deforestation from this relocated population represents a real addition to deforestation emissions. Newly-arrived settlers on the Transamazon Highway near Altamira (Pará) cleared forest at an annual rate averaging 3.6 ha per family during the first five years, while in Ouro Preto do Oeste (Rondônia), lots were cleared at an average annual rate of 2.7 ha per family during the first six years the lot was occupied, after which the clearing rate fell to very low levels until the lot was sold to a new owner (Fearnside 1984). Logging activity that would have continued in the submergence area had the reservoir not been created was probably displaced to forests outside the submergence area, without representing a net change.
One must also deduct the CH4 emissions that would have been produced by the water in the natural river within the stretch flooded by the reservoir. In the same way, emissions of both CH4 and N2O must be deducted from the seasonally-flooded area (várzea) during the flooding and exposure stages of the river's natural hydrological regime.
Indirect emissions. Known as leakage in discussions over the net benefits of silvicultural plantations as a global warming mitigation measure, indirect effects can substantially increase global warming impacts of a development project, including a hydroelectric dam like Tucuruí. The reservoir made it necessary to relocate a 120-km stretch of the Transamazon Highway, placing this (and its associated feeder roads) in a forested area. Tucuruí displaced 3350 families (17 319 people) according to estimates made after the reservoir had been filled (Monosowski 1990, p. 32). Although some of these people moved to towns, most were moved to settlement areas where they cleared land for agricultural plots, particularly in Gleba Parakanã on the western shore). A severe infestation of Mansonia mosquitoes at this site (Tadei et al. 1991) has caused many of these people subsequently to move to a new area of forest, where additional deforestation was effected. Other people have been attracted to the area by the project and its infrastructure, and have cleared additional forest.
Not all of the emissions from clearing by the population attracted to the dam can be blamed on the project, however, as many of these people would have been clearing forest elsewhere in Amazonia were it not for the dam. The same applies to emissions from urban centres that have grown as a result of the dam. Replacing urban infrastructure flooded by the dam, however, represents a direct impact. Tucuruí flooded the town of Jacundá, requiring complete rebuilding of the town at a new site (Mougeot 1990).
A substantial effect of Tucuruí, either positive or negative, is its role in river transportation. The dam could have greatly facilitated transportation from its catchment area if shipping locks had been completed. The Tocantins River formerly had rapids in the stretch of river now submerged by the reservoir. To bypass the rapids during Brazil's rubber boom, this barrier had motivated construction in 1905 of a railway which is now abandoned.
In 1979, the decision was made that, simultaneously with construction of the Tucuruí Dam, shipping locks would be built (Pinto 1982, p. 47). Although construction of shipping locks was begun, they were abandoned before completion. Barge transport on the Tocantins River would be a less energy-consuming means of exporting ore from the Carajás mine than the railway option later adopted. Carajás has the world's largest high-grade iron ore deposit, with an estimated 11 ´ 109 t deposit of ore, which is sufficient for mining at the current rate for 400 years. In addition to iron, the Carajás area has minable deposits of copper, bauxite and other minerals, and is associated with the Grande Carajás Program to administer an agricultural plan eventually expected to export large amounts of soybeans and other agricultural products (see Fearnside 1986, 1989a). Ore and other products from Carajás are now exported using a 890-km railway which was completed in 1983. With the railway a fait accompli, finishing the locks at Tucuruí came to be viewed as part of a second phase of development of Carajás to allow expansion of exports beyond the limits imposed by the railway's capacity (Pinto 1982, p. 46). It is also an option for substantially increasing agricultural exports from the Tocantins-Araguaia Basin, especially of soybeans. Should the locks be completed, then the reservoir would begin to yield an energy and carbon savings by avoiding fuel use for additional rail transport.
The capacity of the railway (30 million t y-1 of ore) limits exports, which might be larger today were transportation done by barge. Because ore at Carajás is extraordinarily pure (66% iron), the mining of lower grade deposits elsewhere in the world (including those in Minas Gerais, Brazil), results in more GHG emissions from transportation and smelting than would be the case were this iron being supplied from Carajás.
Some possibility exists that the locks in the Tucuruí Dam may one day be completed. Although the subject is periodically raised, no specific commitment has been made, and it is therefore more realistic to calculate emissions scenarios without these facilities.
Standardization of level of service. Comparing different types of energy generation requires decisions concerning the level of service, that is, the constancy of electricity supply, that must be supplied by each. Hydroelectric generation in Amazonia has a strong seasonal cycle of energy supply due to seasonal availability of water for power generation. Were electricity to be offered at the same level of service by both hydro and thermal options, for comparative purposes, one would have to include emissions of the backup thermoelectric generators necessary to supply power at the full rate throughout the year. Standardizing level of service is an accepted technique for comparing options that differ greatly in power reliability, as in the case of wind power and thermal generation.
In the case of Tucuruí, a complete standardization at the peak power might be unrealistic as a representation of the real choices involved. When the services being supplied are essential, as in supplying urban centers, thermal backup is needed during low-water periods. For example, Balbina (supplying Manaus, Amazonas) is completely backed up by thermal plants. In the case of Tucuruí, however, about two-thirds of the power is used for aluminium manufacture, and it may be more economical to only smelt aluminium in proportion to available hydropower rather than to supply large amounts of more expensive supplementary power from thermal backup systems. In any case, some form of correction for thermal backup, either full or partial, is needed for a fair comparison of Tucuruí with thermal generation.
Time and the impacts of hydroelectric versus fossil fuel
Timing of emissions. The initial pulse of emissions when a reservoir is flooded, particularly from CO2 released from decay of dead trees projecting above the water, greatly exceeds the dam's global warming benefits in terms of fossil fuel substitution. Different dams vary tremendously in the time required to break even on an instantaneous basis, that is, for annual emissions to fall to a level below that required to produce the same power from fossil fuel, omitting the accumulated impacts of the initial peak of emissions.
Methane emissions calculated from the present assumptions are almost constant over time. However, there is some evidence that a much greater pulse of CH4 is emitted soon after the reservoir is filled. Tundisi (unpublished; see Rosa et al. 1996c, pp. 144 and 150) measured CH4 emissions with floating chambers at Samuel 0.25 y after flooding and at Tucuruí 4.5 y after flooding; these measurements have been used by Rosa et al. (1996c, pp. 148-149) to estimate flux rates of methane from the reservoir surface of 227 g C m-2 y-1 at Samuel and 0 at Tucuruí. The lack of CH4 emissions at the water surface at Tucuruí does not mean that the reservoir was not emitting this gas through other means, especially from water passing through the turbines.
Rationale for discounting. The difference between the importance of a tonne of GHG in the atmosphere now versus 15 years from now is the impacts of global warming caused by those gases over 15 years, such as floods and droughts. How much value society places on these impacts is a major factor in evaluating hydroelectric contributions to global warming.
Rosa & Schaeffer (1994) have proposed an alternative to the IPCC's global warming potentials such that the timing of emissions is considered, and the radiative forcing impact is only counted from the time of emissions onward. This is an advance over the IPCC method, which is based on comparison of simultaneous emission of a molecule of CO2 and a molecule of each other gas, such as CH4. The Rosa & Schaeffer (1994) method does not, however, include any weighting for time preference, assuming a discount rate of zero. Both features are needed: consideration of the timing of the radiative forcing (i.e. the timing of the presence of the atmospheric load of gases, as distinct from the timing of the emissions), and consideration of the weight society gives to time. Rosa & Schaeffer's (1994) method also differs from the one adopted here in having different time horizons over which emissions are considered and over which the radiative effects of the atmospheric loads are considered. In the method adopted here, both end at a common point in time (100 years after closing the dam).
Both the Rosa & Schaeffer (1994) formulation and the one adopted here imply considering the different gases in a way different from that currently adopted by the IPCC. The principal justification for the GWP formulation of IPCC is that a more complicated formulation would be too difficult for policy-makers to understand (R.T. Watson, public statement 1992). However, GWPs are, in fact, a black box from the point of view of decision-makers, who do not grasp the details of how GWPs are derived. Under such circumstances we may as well use a more complicated formulation that better reflects the importance of timing of emissions and of their impacts on radiative forcing. The new black box would be used in the same way by decision-makers, and the result would be a fairer comparison of energy options in terms of social interests.
Effects of time preference. The question of applying discounting (or an alternative time weighting) to GHG emissions and/or their impacts is a matter of debate. The Global Environment Facility (GEF), which administers World Bank funds intended for combating global warming under Agenda 21, currently does not discount carbon or GHGs and their impacts.
Sound reasons exist for some form of time preference weighting for global warming impacts, rather than a zero-discount scheme. Buildup of GHGs in the atmosphere initiates a stream of impacts (including increases in human death rates), not just single-event impacts. If this stream of impacts begins later rather than sooner, the savings (human lives, for example) between the sooner and the later time represent a permanent gain, even though the same individuals may die the next year. The logic is directly parallel to the accepted practice of considering avoided fossil fuel emissions as permanent savings, even though the same barrel of oil may be burned the next year. Applying even a very small discounting would greatly increase the impact of the large initial pulse of hydroelectric emissions relative to the evenly distributed emissions from fossil fuel.
The long atmospheric life of some GHGs, particularly the 125-year average life of CO2 (Albritton et al. 1995), means that global-warming impacts continue long after an emission occurs. Even were emissions to be greatly reduced, the atmospheric load remaining from past emissions would continue to provoke droughts, floods, and other impacts. These features of climatic change contribute to the rationale for some form of discounting or other time preference weighting.
Although not addressed specifically, discounting is implicit in the FCCC's emphasis on annual balance of net emissions, implying that this will be the criterion for any penalties later negotiated as protocols under the Convention. This is implied by the agreement of all countries to conduct inventories of the annual fluxes of emissions (as opposed, for example, to net committed emissions, which would capture the long-term differences between hydroelectric and thermoelectric generation). The annual balance criterion implies discounting because the countries of the world do, in fact, apply discounting when considering money. This means that, from the point of view of national planning, financial costs of the climatic impacts, financial costs of mitigating measures, and financial costs of any fines or taxes on emissions would be treated this way.
Among the implications of the annual balance criterion (and therefore discounting) is that delay in negotiating protocols to implant fines and similar measures creates a motivation to build hydroelectric projects now rather than later. In this way the large pulse of emissions is not counted against the country's annual balance of net emissions.
Assuming that a discount rate greater than zero is applied, the value chosen would have a great influence on the energy choices indicated as preferable. Just as with the case of discounting for financial calculations, hydroelectric dams will be indicated as more attractive than thermal generation if lower discount rates are used. In financial calculations, proponents of hydroelectric dams usually argue strongly for lower discount rates than those used for other kinds of investments. Because of the long lag times between financial investments and the initiation of revenue from electricity sales, hydroelectric development would often be unattractive at the higher discount rates. Hydroelectric proponents do not, however, argue for zero discount rates in the financial sphere. The same needs to be applied to the benefits and impacts in the global warming sphere.
According to some, the discount rate used for carbon must be the same as the one used for money (B. Solberg, public statement 1994). The discount rate used for carbon might be different (i.e. lower) than that used for money (D. Ahuja, personal communication 1992); previous work on global warming potentials has used an annual discount rate of 5% (Lashof & Ahuja 1990).
Discount rates of 10-12% are common in financial analyses of major development projects in Amazonia. Some World Bank economists have even recommended using discount rates of 15% for projects in Brazilian Amazonia (Skillings & Tcheyan 1979). These and other discount rates represent adjustments of real value, that is, after correction for inflation. My preference (Fearnside, unpublished manuscript) under an alternative time preference scheme has an integral effect (the area under the time weighting curve) equivalent to an annual discount rate of 1.24%.
Discount rates in the range used for financial calculations would have a dramatic effect on the attractiveness of hydroelectric generation from a global warming perspective (Fig. 6). At a 15% annual discount rate, fossil fuel becomes more attractive in the case of the current calculations for Tucuruí. The discount rate at which this turnover would occur would be lower were a proper accounting made of many of Tucuruí's emissions (construction, displaced deforestation, etc.). It should also be remembered that Tucuruí is better than the average dam.
Discounting would have a significant effect on the importance given to emissions from the construction phase of hydroelectric dams, such as those from the concrete, steel and transport of materials. Because these emissions occur before the dams are closed, the year of emission is negative and their impact will have to be inflated, rather than deflated, to standardize them to year zero. As compared to fossil fuels, the long lead time of hydroelectric dam construction, as well as the greater requirements for materials, will make this factor weigh against hydro.
Plans for expansion and basin development
The present calculation has considered only the present (Tucuruí-I) configuration of the dam. Further increases in output (and in impacts) may occur in the future were the normal operating level increased to allow installation of more turbines. Output and impacts may also increase by construction of additional dams upstream of the first, thereby regulating the flow of the river in order to provide more water during the low-water season. The additional impacts of raising the water level and/or building additional dams would, of course, have to be taken into account.
ELETRONORTE has plans to expand the installed capacity of Tucuruí to 7960 MW in the Tucuruí-II project. ELETRONORTE has, to this author's knowledge, never released a figure for the area of the Turucuí Reservoir at an elevation 74 m above mean sea level, the normal operating level for the Tucuruí-II project in the original plan for this addition. The result has been that a number of authors (including this one) have calculated energy density values for the full configuration of Tucuruí-II, using the area at the 72 m normal operating level adopted for the now-installed Tucuruí-I configuration of 3960 MW (e.g. Fearnside 1989b; Goodland 1980). ELETRONORTE has since decided that the settlers who have moved into the area along the present shoreline would make raising the water level to 74 m politically impossible, and the current plans for Tucuruí-II call for maintaining the water level at 72 m (John Denys Cadman, personal communication 1996). It is not known how much, if any, the change would reduce the amount of power that the Tucuruí-II configuration could produce annually.
An indication of the additional area that would be flooded were the water level to be raised to 74 m for Tucuruí-II is given by a survey of vegetation, which considered 415.37 km2 to be in the area expected to be flooded in the second stage (Revilla Cardenas et al. 1982). Using the LANDSAT-measured area for Tucuruí-I, we can calculate that the total surface area of the reservoir if the water level of Tucuruí-II is raised would be 2662 km2. If areas used in the vegetation survey are used, the total area (forest and riverbed) would be 3047 km2.
In addition to any further flooding of area of Tucuruí, the Tucuruí-II scheme would require regulation of the flow of the Tocantins River by means of the construction of the Santa Isabel Dam on the Araguaia River, the first major tributary upstream of Tucuruí (Paulo Edgar Dias Almeida, personal communication 1991). The impacts of this must therefore be considered in evaluating the Tucuruí-II proposal. ELETRONORTE has plans to build dams upstream of Tucuruí on the Tocantins and Araguaia rivers (see Junk & de Mello 1987, p. 370). The impacts and benefits of these more extensive schemes would have to be evaluated together with Tucuruí. The full plan for development of the Tocantins/Araguaia basin calls for 26 dams upstream of Tucuruí.
CONCLUSIONS
The Tucuruí Dam produces significant emissions of greenhouse gases, although less would be produced by fossil fuels when considered over a 100-year time horizon. The relative attractiveness of hydroelectric versus thermoelectric generation, in terms of global warming impact, is highly sensitive to discount rate or other forms of weighting for time preference. This author views the nature of climatic change impacts as making some form of time preference weighting appropriate. The global warming impacts of Tucuruí can even exceed those of fossil fuel generation if assessed using discount rates common in financial analyses (rates that fall within the range of discussion on the issue, although not recommended by this author). Because the ratio of energy benefits to global warming impacts at Tucuruí is more favourable than with the average existing dam, or the average planned dam in Brazilian Amazonia, decisions regarding discounting of global warming impacts will be critical to the choices to be made among energy options in the region.
APPENDIX: TUCURUÍ DEPTH AND AREA
Tucuruí's area at its normal pool level of 72 m above mean sea level is officially stated to be 2430 km2 (ELETRONORTE no date [1987], pp. 24-25), which is close to the 2247 km2 area in 1989 measured using LANDSAT-TM imagery (Fearnside 1995) that is used in the calculations that follow. ELETRONORTE, Brazil's state power monopoly for northern Brazil, built and operates the Tucuruí Dam. The riverbed area was 321 km2, considering a reservoir length of 170 km and an average width of 1891 m estimated by me from 1:1 000 000-scale side-looking airborne radar (SLAR) imagery (Projeto RADAMBRASIL 1981). Considering the LANDSAT-measured water surface area, minus the riverbed area and the previously deforested area (ignoring any pre-flooding deforestation that was not done by ELETRONORTE), the area of forest lost to flooding was 1926 km2. The 100 km2 cleared by ELETRONORTE in the reservoir area also resulted in greenhouse-gas emissions of c. 20 000 t C, which are not considered here. Reservoir filling lasted from 6 September 1984 to 20 March 1985.
The drawdown depth is 14 m (ELETRONORTE no date [1992], p. 5), and the average depth of the reservoir at the minimum water level can be calculated to be 9.7 m. This average depth is based on a minimum normal operating level of 58.0 m above mean sea level (ELETRONORTE no date [c.1983]). A minimum operating level of 51.6 m (ELETRONORTE no date, p. 2‑1; ELETRONORTE no date [1992]) implies a drawdown depth of only 3.3 m. Forest area flooded at minimum water level is taken as proportional to water volumes at these two levels from ELETRONORTE (no date [c.1983], p. 6).
The area of forest flooded at the operating level is 192 553 ha, and at the minimum water level is 106 787 ha (Fearnside 1995, Table V). Of the area cleared prior to flooding, 8000 ha is assumed to be in the permanently flooded zone and 2000 ha in the seasonally flooded zone.
ACKNOWLEDGEMENT
This paper is abbreviated from a presentation at the International Workshop on Greenhouse Gas Emissions from Hydroelectric Reservoirs, Rio de Janeiro, Brazil, 11-12 September 1996. An earlier version was presented at the International Atomic Energy Agency (IAEA) Advisory Group Meeting on Assessment of Greenhouse Gas Emissions from the Full Energy Chain in Hydropower, Nuclear Power and other Energy Sources, 12-14 March 1996, Montreal, Quebec, Canada. I thank S.V. Wilson and two anonymous reviewers for comments.
REFERENCES
Albritton, D.L., Derwent, R.G., Isaksen, I.S.A., Lal, M. & Wuebbles, D.J. (1995) Trace gas radiative forcing indices. In: Climate Change 1994: Radiative Forcing of Climate Change and An Evaluation of the IPCC IS92 Emission Scenarios, ed. J.T. Houghton, L.G. Meira Filho, J. Bruce, Hoesung Lee, B.A. Callander, E. Haites, N. Harris & K. Maskell, pp. 205-31. Cambridge, UK: Cambridge University Press: 339 pp.
Baxter, R.M. (1977) Environmental effects of dams and impoundments. Annual Review of Ecology and Systematics 8: 255-83.
Buschbacher, R.J. (1984) Changes in Productivity and Nutrient Cycling following Conversion of Amazon Rainforest to Pasture. Ph.D. dissertation in ecology. Athens, Georgia, USA: University of Georgia: xiii + 193 pp.
ELETRONORTE (no date [1992]) Ambiente
Desenvolvimento Tucuruí. Brasília, Brazil: Centrais Elétricas do Norte do
Brasil (ELETRONORTE): 32 pp.
ELETRONORTE (no date [c.1983])
Usina Hidrelétrica Tucuruí 8.000 MW. Brasília, Brazil: Centrais
Elétricas do Norte do Brasil (ELETRONORTE): 27 pp.
ELETRONORTE (no date [1987]) Livro
Branco sobre o Meio Ambiente da Usina Hidrelétrica de Tucuruí. Brasília,
Brazil: Centrais Elétricas do Norte do Brasil (ELETRONORTE): 288 pp.
ELETRONORTE (no date) UHE
Tucuruí: Plano de Utilização do Reservatório, Caracterização e Diagnóstico do
Reservatório e de sua Área de Influência. TUC-10-263-46-RE Volume 1 - Texto. Brasília, Brazil: Centrais Elétricas do Norte
do Brasil (ELETRONORTE): Irregular pagination.
ELETRONORTE/MONASA/ENGE‑RIO
(1976) Estudos Amazônia, Relatório Final Volume IV: Aproveitamento
Hidrelétrico do Rio Uatumã em Cachoeira Balbina, Estudos de Viabilidade.
Brasília, Brazil: Centrais Elétricas do Norte do Brasil (ELETRONORTE)/ MONASA
Consultoria e Projetos Ltda./ENGE-RIO Engenharia e Consultoria: Irregular
pagination.
Fearnside, P.M. (1984) Land clearing behaviour in small farmer settlement schemes in the Brazilian Amazon and its relation to human carrying capacity. In: Tropical Rain Forest: The Leeds Symposium, ed. A.C. Chadwick & S.L. Sutton, pp. 255-71. Leeds, UK: Leeds Philosophical and Literary Society: 335 pp.
Fearnside, P.M. (1986) Agricultural Plans for Brazil's Grande Carajás Program: Lost Opportunity for Sustainable Development? World Development 14: 385-409.
Fearnside, P.M. (1989a) The charcoal of Carajás: Pig‑iron smelting threatens the forests of Brazil's Eastern Amazon Region. Ambio 18: 141-3.
Fearnside, P.M. (1989b) Brazil's Balbina Dam: Environment versus the legacy of the pharoahs in Amazonia. Environmental Management 13: 401-23.
Fearnside, P.M. (1990) Environmental destruction in the Brazilian Amazon. In: The Future of Amazonia: Destruction or Sustainable Development? ed. D. Goodman & A. Hall, pp. 179-225. London: Macmillan: 419 pp.
Fearnside, P.M. (1995) Hydroelectric dams in the Brazilian Amazon as sources of 'greenhouse' gases. Environmental Conservation 22: 7-19.
Fearnside, P.M. (1996a) Amazonia and global warming: Annual balance of greenhouse gas emissions from land-use change in Brazil's Amazon region. In: Biomass Burning and Global Change. Volume 2: Biomass Burning in South America, Southeast Asia and Temperate and Boreal Ecosystems and the Oil Fires of Kuwait ed. J. Levine. pp. 606-617. Cambridge, Massachusetts, USA: MIT Press: 902 pp.
Fearnside, P.M. (1996b) Hydroelectric dams in Brazilian Amazonia: Response to Rosa, Schaeffer & dos Santos. Environmental Conservation 23(2): 105-108.
Goodland, R.J.A. (1980) Environmental ranking of Amazonian development projects in Brazil. Environmental Conservation 7: 9-26.
Grace, J.,
Lloyd, J., McIntyre, J., Miranda, A.C., Meir, P., Miranda, H.S., Nobre, C.,
Moncrieff, J., Massheder, J., Malhi, Y., Wright, I. & Gash, J. (1995)
Carbon dioxide uptake by an undisturbed tropical rain forest in southwest
Amazonia, 1992 to 1993. Science 270: 778-80.
Junk, W.J. & de Mello, J.A.N. (1987) Impactos ecológicos das represas hidrelétricas na bacia amazônica brasileira. In: Homem e Natureza na Amazônia, ed. G. Kohlhepp & A. Schrader, pp. 367‑85. Tübinger Geographische Studien 95 (Tübinger Beiträge zur Geographischen Lateinamerika-Forschung 3). Tübingen, Germany: Geographisches Institut, Universität Tübingen: 507 pp.
Keller, M., Kaplan, W.A. & Wofsy, S.C. (1986) Emissions of N2O, CH4 and CO2 from tropical forest soils. Journal of Geophysical Research (Atmospheres) 91: 11 791-802.
Klinge, H. & Rodrigues, W.A. (1973) Biomass estimation in a central Amazonian rain forest. Acta Cientifica Venezolana 24: 225-37.
Lashof, D.A. & Ahuja, D.R. (1990) Relative global warming potentials of greenhouse gas emissions. Nature 344: 529-31.
Leentvaar, P. (1966) The Brokopondo research project, Suriname. In: Man-Made Lakes, ed. R.H. Lowe-McConnell. London: Academic Press: 218 pp.
Lovejoy, T.E., Rankin, J.M., Bierregaard, Jr., R.O., Brown, Jr., K.S., Emmons, L.H. & van der Voort, M.E. (1984) Ecosystem decay of Amazon forest remnants. In: Extinctions, ed. M.H. Nitecki, pp. 295‑325. Chicago, Illinois, USA: University of Chicago Press.
Martius, C., Fearnside, P.M., Bandeira, A.G. & Wassmann, R. (1996) Deforestation and methane release from termites in Amazonia. Chemosphere 33: 517-36.
Martius, C., Wassmann, R., Thein, U., Bandeira, A.G., Rennenberg, H., Junk, W.J. & Seiler, W. (1993) Methane emission from wood-feeding termites in Amazonia. Chemosphere 26(1-4): 623-32.
Monosowski, E. (1990) Lessons from the Tucuruí experience. Water Power and Dam Construction February 1990: 29-34.
Mougeot, L.J.A. (1990) Future hydroelectric development in Brazilian Amazonia: Towards comprehensive population resettlement. In: The Future of Amazonia: Destruction or Sustainable Development? ed. D. Goodman & A. Hall, pp. 90-129. London: MacMillan: 419 pp.
Novo, E.L.M.
& Tundisi, J.G. (1994) Contribution of remote sensing techniques to the
assessment of methane emission from large tropical reservoirs. Remote Sensing Reviews 10: 143-53.
Revilla Cardenas, J.D., Kahn, F.L.
& Guillamet, J.L. (1982) Estimativa da fitomassa do reservatório da UHE de
Tucuruí. In: Projeto Tucuruí, Relatório Semestral, Período janeiro/junho
1982, Vol. 2: Limnologia, Macrófitas, Fitomassa, Degradação da Fitomassa,
Doenças Endêmicas, Solos, pp. 1-11. Manaus, Amazonas, Brazil: Centrais
Elétricas do Norte do Brasil (ELETRONORTE) & Instituto Nacional de
Pesquisas da Amazônia (INPA): 32 pp.
Pereira, A. (1989) Étude des données limnologiques
de la retenue de Tucuruí, Amazonie-Brésil. Rapport de Stage de D.E.A.
Techniques et Gestion de l'Environnement. Cayenne, French Guiana: CERGRENE-ENPC.
Pinto, L.F. (1982) Carajás, O
Ataque ao Coração da Amazônia, 2a. edição. Rio de
Janeiro, Brazil: Editora Marca Zero: 140 pp.
Projeto RADAMBRASIL (1981) Mosaico
semi-controlado de Radar. Map Scale: 1:250,000. Folhas SA-22-ZC, SB-22-XA,
SB-22-XB and SB-22-SD. Rio de Janeiro, Brazil: Departamento Nacional de
Produção Mineral (DNPM).
Rosa, L.P. & Schaeffer, R. (1994) Greenhouse gas emissions from hydroelectric reservoirs. Ambio 23: 164-5.
Rosa, L.P. & Schaeffer, R. (1995) Global warming potentials: The case of emissions from dams. Energy Policy 23: 149-58.
Rosa, L.P.,
Schaeffer, R. & dos Santos, M.A. (1996a) A Model of Greenhouse
Gas Emissions from Hydroelectric Plants and an Application to Dams in the
Amazon Region of Brazil. Rio
de Janeiro, Brazil: Coordenação dos Programs de Pós-Graduação de Engenharia
(COPPE), Universidade Federal do Rio de Janeiro (UFRJ): 17 pp.
Rosa, L.P.,
Schaeffer, R. & dos Santos, M.A. (1996b) Are hydroelectric dams in
the Brazilian Amazon significant sources of 'greenhouse' gases? Environmental Conservation 23: 2-6.
Rosa, L.P., Schaeffer, R. & dos
Santos, M.A. (1996c) Emissões de metano e dióxido de carbono de
hidrelétricas na Amazônia comparadas às termelétricas equivalentes. Cadernos de Energia No. 9: 109-157
Roulet, M. (1992) Environnement des Barrages
Hydroélectriques: Analyse du Projet d'Aménagement Amazonien de Petit-Saut en
Guyane Française. Montreal, Canada: Rapport de Stage de la maîtrise en
Sciences de l'Environnement, Université du Québec à Montreal: 113 pp.
Skillings, R.F. & Tcheyan, N.O. (1979) Economic development prospects of the Amazon Region of Brazil. Baltimore, Maryland, USA: Center of Brazilian Studies, School of Advanced International Studies, Johns Hopkins University, Occasional Paper No. 9.
Tadei, W.P., Scarpassa, V.M. &
Rodrigues, I.B. (1991) Evolução das populações de Anopheles e de Mansonia,
na área de influência da Usina Hidrelétrica de Tucuruí (Pará). Ciência e
Cultura 43(7) suplemento:
639-40.
FIGURE LEGENDS
Figure 1 Brazil's Legal Amazon Region.
Figure 2 Tucuruí emissions: (A) from the hydroelectric project, (B) from fossil fuel displaced by Tucuruí.
Figure 3 Tucuruí: net greenhouse-gas emissions.
Figure 4 Tucuruí: atmospheric loads of greenhouse gases (A) from the hydroelectric project, (B) from fossil fuel displaced by Tucuruí.
Figure 5 Tucuruí: radiative forcing of the atmospheric loads of greenhouse gases (A) from the hydroelectric project, (B) from fossil fuel displaced by Tucuruí. The centre of gravity of the total radiative forcing curve is at year 52 for hydroelectric emissions and at year 67 for fossil fuel emisions, a difference of 15 years.
Figure 6 Tucuruí:
discounting greenhouse gas benefits and impacts (global warming benefit/impact
ratios).
Fig. 2
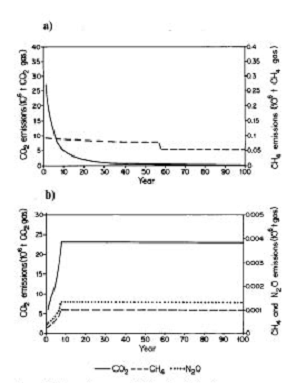
Fig. 3
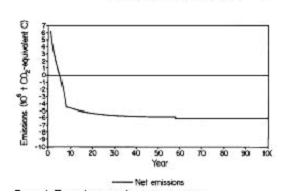
Fig 4A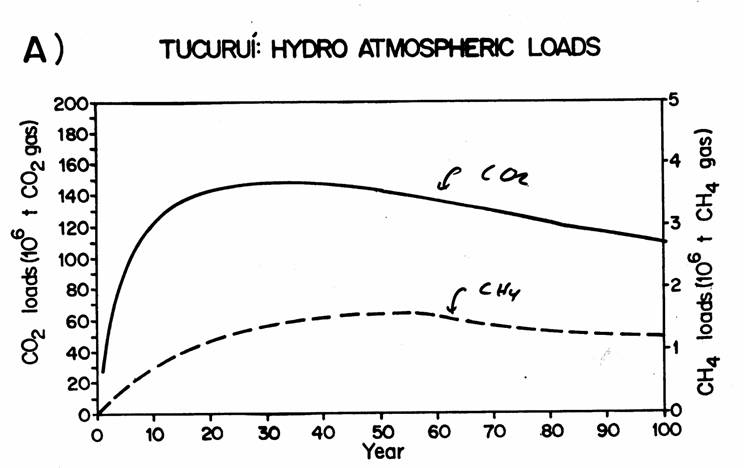
Fig. 4B
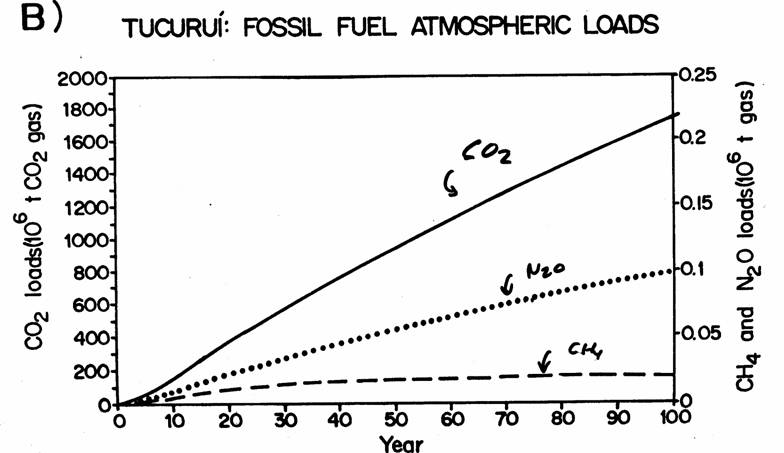
Fig. 5
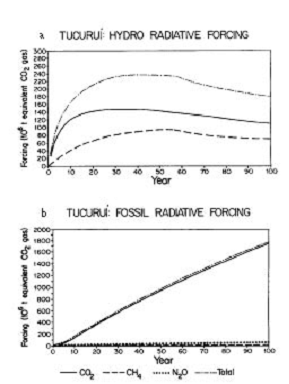
Fig 6
