The
text that follows is a PREPRINT.
Please cite as:
Nogueira, E.M., B.W. Nelson and P.M. Fearnside. 2005. Wood density
in dense forest in central Amazonia, Brazil. Forest Ecology and Management 208(1-3): 261-286.
ISSN:
0378-1127
Copyright:
Elsevier
The
original publication is available at: http://www.elsevier.com.nl
Wood density in dense forest
in central Amazonia, Brazil
Euler
Melo Nogueiraa,
Bruce Walker Nelsonb, Philip M. Fearnsideb
aGraduate Program in Tropical
Forest Science, National Institute for Research in the Amazon – INPA, C.P. 478,
69.011-970 Manaus, Amazonas, Brazil
bDepartment of Ecology,
National Institute for Research in the Amazon – INPA,
C.P. 478, 69.011-970 Manaus, Amazonas, Brazil
13 July 2004,
revised 1 Dec. 2004
Address
for proofs:
Philip
M. Fearnside
INPA
Av. André
Araújo, 2936
C.P. 478
69.011-970
Manaus, Amazonas
Brazil
tel:
+55-92-643-1822
fax.
+55-92-642-8909
email: pmfearn@inpa.gov.br
abstract :400 words, main text : 6108 words
In press: Forest Ecology and Management
Abstract
Measurements
of wood density of trees in Amazonian forests are necessary to reduce
uncertainties in estimates of carbon stocks and of greenhouse-gas emissions
from deforestation. Based on samples from 310 trees in 186 species or
morpho-species collected near Manaus, Brazil, the present study finds that
commonly used wood density estimates found in published lists by species need
to be adjusted downward by 5.3%. Taking the average bole density from this
study as a standard, wood density overestimations in three prior studies of the
central Amazon were found to be 6%, 4% and 0%. Estimates of primary forest
biomass and of gross emissions from biomass loss through deforestation will
have to be reduced by similar percentages. Considering full disks with bark
dried at 103ºC, the mean basic density at breast height in the Central Amazon
dense forest was 0.704 ± 0.117 (mean ± 1 standard deviation; n=310; range
0.27-0.96); at the top of the bole it was 0.647 ± 0.093 (n=307; range
0.26-0.87). The arithmetic mean of the basic density of the trunk -- average of
the density at breast height and at the top of the bole -- was 0.675 ± 0.101
(n=307; range 0.27-0.91). The mean basic density of the bole, adjusted for
tapering, and using four samples along the bole, was 0.670 ± 0.099 (n=71; range
0.38-0.86). The arithmetic mean of the basic density for the same trees was
0.675 ± 0.098 (range 0.39-0.87). The basic density of central heartwood was
0.766 ± 0.158 (n=149; range 0.34-1.06). Significant differences exist between
the various published estimates for Amazonian forest biomass and emissions, but
we emphasize that revision of density values based on the present study will
not reduce these discrepancies; instead, all estimates will shift in parallel
to lower levels. Adjustments to biomass and emissions are sufficiently large to
be significant for the global carbon balance. For example, an estimate of net
committed emissions of 249 × 106 Mg CO2-equivalent
C/year for Brazilian Amazonia in the 1990, of which 237 × 106 Mg CO2-equivalent
C/year was from net removal of biomass, would be reduced by 14 × 106 Mg
CO2-equivalent
C/year (5.7%: larger than the 5.3% adjustment to gross emissions because
regrowth estimates remain unchanged). Decreases of similar proportions would
apply throughout the tropics. For the 1980s these downward adjustments total
113 × 106 Mg
C/year for CO2 effects alone, or
approximately 132 Mg CO2-equivalent C/year including trace gases.
Keywords: Amazon forest, Basic density, Wood density,
Basic specific gravity.
1.
Introduction
Greenhouse gas
emissions from tropical deforestation represent one of the largest
uncertainties concerning global climate change (Houghton, 2003). Emissions when
forests are cleared are almost directly proportional to the biomass (including
both live and dead material) of the forest, which, in turn, depends on the
volume of wood and its density. Because such large amounts of biomass are
cleared each year, even small alterations in estimates of wood density
translate into quantities for forest biomass and greenhouse gas emissions that
are significant for global change.
Density is an important
physical characteristic of wood in defining technological and commercial use
because it is an excellent indicator of the amount of wood present in a sample
and of the workability of the material (Silva, 1984; Trugilho et al., 1990;
Chimelo, 1992; ASTM, 2002). The density is related to other properties of the
wood, such as resistance, porosity, organization of the anatomical components
and the number, size, and chemical composition of the cells (Kollmann and Côté,
1968; Trugilho et al., 1990; Simpson and TenWolde, 1999; Ilic et al., 2000;
Hacke et al., 2001; ASTM, 2002). In tropical forests wood density is related to
a tree’s resistance to impacts caused by wind, to relative growth rate and to
mortality (Putz et al., 1983; Muller-Landau, 2004). Wood density is also a
strong indicator of the stage of ecological succession, with pioneer species
being less dense and having greater variation than climax species (Denslow,
1980; Wiemann and Williamson, 1989; Muller-Landau, 2004). There is great
variation in density along the bole, among species, and among individuals in
any given species due to differences in the age of the tree and in the climatic
life zone (Chudnoff, 1976; Wiemann and Williamson, 1989, 2002; Rueda and
Williamson, 1992; de Castro et al., 1993; Rocha, 1994; Higuchi et al., 1998;
Woodcock, 2000; Baker et al., 2004; Muller-Landau, 2004). Variation has been
observed from the heartwood to the bark, along the length of the bole (the
trunk below the first large branch), among different compartments in a given
tree and between individuals of the same species. This variation reflects the
interaction of the plant with environmental factors such as climatic and
edaphic conditions, natural impacts and competition for light (Chudnoff, 1976;
Wiemann and Williamson, 1989; Trugilho et al., 1990; Ilic et al., 2000; França,
2002; Muller-Landau, 2004).
Different methodologies have
been used for determining the weight and volume measures, the ratio of which
represents density, resulting in different concepts (Trugilho et al., 1990;
Fearnside, 1997a). Weight has been determined with different moisture contents,
with volume either with or without bark, and using volume either of the fresh
wood, of dry wood, or of wood that has been dried and later re-hydrated. Among
the different ways of calculating density are apparent density (the ratio
between weight and volume at a given moisture content), green density (green
weight/green volume), simple specific gravity (dry weight/dry volume), true
density (excluding naturally occurring pores in the wood by compression of the
sample), and basic density or basic specific gravity, which is obtained as the
ratio between the dry weight and the volume of the green wood (Fearnside,
1997a; Souza et al., 2002). Basic density was used in the present study and is
considered to be the most appropriate density measure for biomass estimation
(Brown, 1997; Fearnside, 1997a).
Based on 470 samples from tropical American forests,
Reyes et al. (1992) found a mean density of 0.60 ± 0.008 g/cm3 (mean
± 1 standard error). Brown and Lugo (1992) report a mean of 0.69 for Amazonia,
based on a relationship between biomass and bole volume from data (diameter,
species and the volume of all trees) reported by Heinsdijk (1958) and Prance et
al. (1976) for two areas of Amazonian forest. Muller-Landau (2004) examined 112
trees from dense forest of the Central Amazon. These represented 89 species and
their density was either determined directly from thin wood cores of the full
xylem radius or was based on published data at the species level. The 89
species constituted 19% of the trees in a nearby large inventory. When weighted
for abundance in that inventory, the 89 species had a mean density of 0.71 ±
0.15 g/cm3 (mean ± 1 standard deviation).
Using
mainly the inventories of RADAMBRASIL (Brazil, Projeto RADAMBRASIL, 1976-1986)
and published lists of density by species (Fearnside, 1997a) the mean basic
density for Brazilian Amazonia was estimated at 0.69, considering the different
vegetation types and their respective areas. For dense lowland forest
in the state of Amazonas, the mean density reported is 0.70 g.cm-3. This value contains uncertainty due to doubts
concerning the taxonomy of the species (names are usually only reliable to the
genus level) and use of density values determined by different methods
(Fearnside, 1997a).
A reliable value for mean
density for forests in Amazonia is necessary so that volumetric estimates
available from extensive inventories can be converted to estimates of biomass
stock (Brown et al., 1989; Brown and Lugo, 1992; Fearnside, 1997a; Houghton et
al., 2001). Mean density has also been used in adapting allometric models
developed for dense forest to make them applicable to other types of forest,
correcting for the effect of density differences (França, 2002; Baker et al.,
2004). Studies of wood density in Amazonia can contribute to reducing
uncertainties in estimates of the stock and emission of carbon, in addition to
contributing to studies of nutrient dynamics in Amazonian ecosystems and to
quantification of forest resources.
The objective of this study
was to determine the basic density of species in dense forest on plateaus with
latosol (Oxisol) soils in central Amazonia, and to evaluate the radial
variation and variation along the length of the bole. The study also determined
the difference between the densities calculated using the volume of re-hydrated
samples and using the fresh volume. A second objective was to evaluate possible
bias toward high or low wood density in previous studies.
2.
Material and Methods
2.1.
Collection site
The collection area is located about 50 km northwest
of Manaus, Amazonas, Brazil, in the Tarumã-Mirim Rural Settlement Project.
Plateau locations were selected in six different lots of small rural farmers.
The area has annual average precipitation of 2075 mm, rainfall below 100 mm per
month from July to September, mean altitude of 100 m, minimum mean monthly temperature of 26ºC and
maximum of 27.6ºC (Brazil, INMET, 2003). The vegetation is dense rain forest of
terra firme (land that is not seasonally flooded), on yellow latosols
(Oxisols) that are poor in nutrients (Magnago et al., 1978; Yamazaki et al.,
1978). Random felling of trees was allowed, this being a new colonization front
(< 5 years) with deforestation for agricultural use already planned and authorized
by the Brazilian Institute for the Environment and Renewable Natural Resources
(IBAMA). The plots selected were under primary forest, without invasion of
pioneer trees or mortality associated with edges.
2.2. Collection of wood samples
Samples of wood of 310 trees
were collected (DBH = 5 to 122 cm) at six different sites distributed over an
area of 45 km2,
sampling approximately 50 trees/site. The collection locations were at least
100 m from the edge of the forest. Trees were chosen to fill quotas for each
size class but otherwise at random. The chain saw operator was not allowed to
choose trees since he might exclude species with very hard wood or with high
silica content, both of which shorten chain life. For all trees disks of
constant thickness were collected at breast height and at the top of the bole
using a chainsaw. For 73 trees, two additional disks were collected at
intermediate points such that all four disks were equally spaced along the
bole. From each of the disks a wedge-shaped sample was removed that was
representative of the radial variations (bark, sapwood and heartwood). Each
wedge was immediately sealed in a plastic bag kept in the shade to avoid loss
of water. Samples of heartwood of 149 trees were also collected at breast height
(~1.36 m). Botanical specimens were collected from every tree for
identification.
2.3. Determination of basic density
On the day each sample was
collected, its volume was determined based on the Archimedes principle by
displacement of water (ASTM, 2002). Impaled with a thin needle, each sample was
forcibly immersed in water in a container resting on a digital balance. The
balance had 2000-g capacity and 1-g precision, and was calibrated daily using a
volumetric flask containing water. The dry weight of each sample was determined
in an oven at 80 and 103oC (ASTM, 2002). A vented electric oven was used in an
air-conditioned room kept at 25oC. Samples, which were kept in
double paper bags, were considered completely dry after three consecutive
stable weight readings, checked every 24 hours. A single tare weight was used
for paper bags from each factory bundle, based on weighing a sheaf of 50 bags
heated to the drying temperature for 24 hours.
2.4. Determination of mean basic density of the bole
Arithmetic mean density of
two or of four measurements along the bole was determined for all 307 trees. A
taper-adjusted mean density was determined for 71 of these trees, which were
sampled at four locations along the bole, using the model of Vital (1984, eq. 1):
Dmb = {Σ (Dms1 * V
seg 1),
(Dms2 * V
seg 2),
(Dms3 * V
seg 3)} *
(Σ Vseg 1, 2 and 3)-1 (eq. 1)
Where:
Dmb = Mean density of the bole,
Dms1 = Mean of the density at breast height,and at 33% of
the length between breast height and the top of the bole,
Dms2 = Mean of the density at 33% and at 66% of the length
between breast height and the top of the bole,
Dms3 = Mean of the density at 66% of the length between
breast height and the top of the bole, and the density at the top of the bole.
Vseg 1, 2 and 3 = Volume of the bole segments at the heights
1.36m - 33%, 33% - 66% and 66% - top, respectively.
The volume of each segment
(the frustum of a paraboloid) was obtained using the Smalian formula:
V = {(Asi + Asf) * 0.5} * h (eq.
2)
Where:
Asi = Cross sectional area at base of segment,
Asf = Cross sectional area at top of the segment,
h
= Length of the segment.
For correct determination of
the area of each cross section of the bole, a drawing was traced of the
external edge of the entire disk, and of the internal edge if the log was
hollow. The drawings were photographed using a digital camera with an 80 mm
lens at a distance of 4 m. The area of each section was determined by counting
pixels later transformed to cm2. Scale varied only 0.6%
between the center and edge of the tracing paper and this was averaged out by
using registration marks at the four corners. When present, the hollow areas
were subtracted in determining the total area of each section. This procedure
was adopted in order to eliminate errors implicit in the common assumption that
the bole is a solid of revolution and that diameter and volume can be inferred
from circumference obtained with a measuring tape. The procedure eliminated
volume overestimates that are caused by the occurrence of trunks with oval
cross-sections, external irregularities above buttresses, or hollow cores;
these conditions are common in Amazonian species.
2.5. Density obtained using re-hydrated volume of
heartwood
Heartwood samples were
always obtained near the center of the disk at breast height, but varied in
size and thus in their surface-to-volume ratios. This will affect re-hydration
rate so three sub-samples were taken, each measuring approximately 2 × 2 × 3 cm
(volume 12 cm3).
To reduce bias in density in the radial direction, the sub-samples were
obtained along the radial axis and a mean density calculated. The sub-samples
were weighed on a digital balance with 0.01 g precision immediately after
drying at 103oC.
They were then immersed in water for 14 days under refrigeration to avoid
decomposition, and the re-hydrated volumes determined by the Archimedes
principle using the same balance.
2.6. Botanical identification
All
botanical samples were identified by experts (parabotanists), who are employees
of the herbarium of the National Institute for Research in the Amazon (INPA).
3.
Results
3.1. Wood density: vertical and radial variation
The 310 trees were
identified as 186 different species or morpho-species, with four trees
unidentified (Appendices 1 and 2). The values for basic density at breast
height and at the upper end of the bole for each species are presented in
Appendix 1. All density values are based on dry weight obtained at 103ºC,
except where noted. Following the classification proposed by Melo et al. (1990), only 5% of
the trees in this study have light wood (density ≤ 0.50 g.cm-3),
64% have wood of medium weight (density 0.50 to 0.72) and 31% have heavy wood
(density > 0.72).
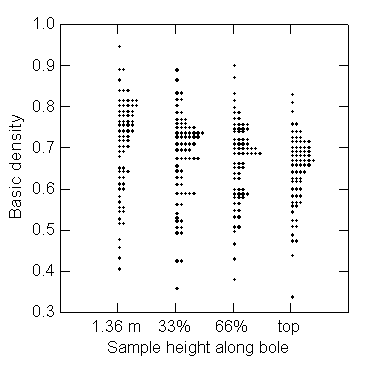
[Figure
1 here]
[Table
1 here]
The mean density generally
decreases from breast height to the top of the bole (Figure 1, Table 1). For 87% of the trees, the density decreased with height on
the bole, the most extreme case being a 57% decrease. Only 13% of the trees
increased in density with height, the most extreme case being a 24% increase.
Density at the top of the bole is 8% lower than at breast height, on average.
Using density of the disk at
breast height as an indication of average density of the entire bole will
result in a 4.3% overestimate of a stand’s average bole wood density. The mean
basic density with bark at breast height for all species was 0.704 ± 0.117
(mean ± 1 standard deviation; n=310; range 0.27-0.96). The mean basic density
with bark at the top of the bole was 0.647 ± 0.093 (n=307; range 0.26-0.87).
The arithmetic mean basic density of the entire bole, based on disks from just
two positions, was 0.675 ± 0.101 (n=307; range 0.27-0.91, Appendix 1),
significantly lower than the density at breast height (paired t-test, p <
0.001, n = 307). For the 73 trees sampled at four positions along the length of
the bole a similar arithmetic mean was obtained: 0.675 ± 0.098 (n=73; range
0.39-0.87). Mean basic density of these trees, adjusted for tapering of the
bole, was similar to the arithmetic mean: 0.670 ± 0.099 (n=71; range
0.38-0.86).
Using
heartwood density at breast height will lead to 5.3% overestimate of density of
the entire disk at that height (paired t-test, p < 0.001, n=149). For the
trees from which heartwood was collected separately, the whole-disk basic
density at breast height was 0.728 on average, while the average density of
just the heartwood at breast height was 0.785 (Figure 2). Not all trees showed
this pattern: for 18% the heartwood density was lower than the full disk by
0-26%. For 80% of the trees the heartwood was 0-20% denser and in 2% of the
trees heartwood was 40-56% denser then the whole disk.
[Figure
2 here]
3.2. Effect of re-hydration and of drying temperature
(80oC
and 103oC)
on density
Using oven-dried samples that
were later re-hydrated to estimate basic density of heartwood led to a 2.5%
overestimate (Table 1; paired t-test, p < 0.001; n=145). The basic density
from green volume of the heartwood, for the trees from which heartwood was collected,
was 0.766 ± 0.158 (range 0.34-1.06). But when obtained using re-hydrated
volume, the density of the heartwood was 0.785 ± 0.167 (range 0.17-1.05).
Fourteen days were insufficient for the complete recovery of the green volume
of small wood blocks of approximately 12 cm3. The difference was
larger with denser wood (p < 0.001; n=144), probably because denser wood is
more resistant to the penetration of
water during immersion. The error in estimating basic density using
re-hydrated samples will therefore probably be less than 2.5% in forest types
or in parts of a tree with basic density lower than 0.766. The error will also
be less if re-hydrating air-dried samples to determine volume prior to oven drying, as is standard
procedure. The widepread practice of re-hydration is undoubtedly due to the
greater convenience of not being obliged to determine volumes immediately after
sample collection.
Density from dry weight at
80oC was, on average, 1.1% higher than at 103oC (Table 1;
paired t-test, p < 0.001, n= 310), despite the dry weight at each
temperature being based on three consecutive stable readings. Although 103oC is
recommended in official protocols for density determination (ASTM, 2002), tests
at 80oC
were conducted as well due to the existence of density data for Amazonia that
were determined at this temperature.
3.3. Relationship of density to morphometric variables
The
arithmetic mean density of the bole showed no significant correlation with bole
height, corrected bole volume or DBH (Figure 3b). But density showed a nearly
significant relationship with total tree height (Figure 3a) ( p = 0.07, Pearson
correlation).
[Figure
3 here]
3.4. Density corrections
In Table 2 simple regressions are presented that allow
estimation of the mean basic density of the entire bole in dense Amazon forest
based on commonly available attributes such as re-hydrated heartwood density,
basic density of heartwood or basic density of the entire disk at breast
height. Basic density of the full disk at breast height is also estimated from
two types of heartwood density at the same height. All models are highly
significant. Residuals are symmetric and non-heteroscedastic.
[Table
2 here]
4. Discussion
4.1.
Causes of density variation within the bole and between species and locations.
In general, studies that have determined radial and
longitudinal variation in density for species in Amazonia (Wiemann and
Williamson, 1989; Amorim, 1991; de Macedo, 1991; de Castro et al., 1993; Higuchi
and Carvalho, 1994), either present results for few species restricted to
certain functional groups, or measure either only radial or only longitudinal
variation. For 145 trees in central Amazonia the present study finds patterns
of radial variation in the dense terra firme forest, with the density
usually decreasing from the center to the outside, at breast height. Therefore,
the portions of the trunk that are more recent have lower density. This result
is in agreement with Fearnside (1997a, Table 1) and Amorim (1991). However, it
is not certain if the same pattern is observed at higher positions of the bole.
Unlike the pattern found here for most of the trees in
dense primary forest, in the case of pioneer tropical species, de Castro et al.
(1993) affirm that density increases linearly from the center to the outside, a
difference that can reach 200-300% in some species. Wiemann and Williamson
(1989) demonstrated for 16 species of tropical trees that density increases
away from the center, the increase being more accentuated (90-270%) in pioneer
species in lowland forest. The same pattern is expected for other colonizing
species. Pioneer species probably allocate resources to growth in stature to
the detriment of the strength of the trunk, resulting in a bole with lower
density and rapid apical growth. In the present study, the density of heartwood
was compared with the density of the
whole disk including bark, unlike the studies of Wiemann and Williamson (1989)
and de Castro et al. (1993), which examined density in 1-2 cm increments along
the radius of the disk at breast height, considering the center of the bole and
not the heartwood. Among the species in the present study, Amapá (Brosimum
parinarioides Ducke (Moraceae)), a canopy tree, had the largest decrease in
density in the center-bark direction, with the heartwood density being 55%
greater than the whole disk with bark. Among the few species that presented the
inverse pattern, the greatest difference (18% density increase in the
center-bark direction) was detected in Sclerolobium melanocarpum Ducke
(Caesalpinioideae), a pioneer emergent tree (Ribeiro et al., 1999).
In the present study, density increased
with the vertical position of the sample in only 14% of the trees. De Macedo
(1991) found that in just one of 12 trees collected near Manaus, the density at
the base of the bole was smaller than in the upper part. These
few cases of lower density at the lower part of the bole may be a consequence
of incipient degradation of the wood, which precedes the formation of a hollow
core. This process would be more advanced close to the base of the tree, where
hollow cores are most common. This could also be responsible for some cases of
lower density of the heartwood found in 18% of the trees when compared with the
density of whole the disk. Cupania scrobiculata L.C. Rich. (Sapindaceae)
had a hollow area occupying 7.6% of the cross section at breast height and also
had lower density of the intact wood at the same height when compared to the
density at the top of the bole. However, no other hollow tree had lower density
at breast height when compared to the top of the bole.
Several authors have pointed to different
ecophysiological aspects as responsible for variation in the density of the
bole, such as structural demands, climatic zone, humidity, age, illumination
and rapid growth (DeZeeuw, 1965; Chudnoff, 1976; Denslow, 1980; Wiemann and
Williamson, 1988; Rueda and Williamson, 1992; de Castro et al., 1993;
Favrichon, 1994; Suzuki, 1999; Ter Steege and Hammond, 2001). Using 56
inventory plots grouped by region, Baker et al. (2004) reported mean
stand-level wood density to be 12% higher in the eastern and central Amazon,
compared with the northwest Amazon. Muller-Landau (2004), analyzing variation
between four widely spread neotropical forest sites, observed that the wood
density varies inversely with the fertility of the soil but is independent of
rainfall, seasonality and temperature. Woodcock (2000) found different mean
wood densities in plots of different successional stages, with lower density in
young successional stages, but did not test for differences among soil types.
Ter Steege and Hammond (2001), in forests in Guyana, failed to find a
relationship between wood density and soil fertility, but did find a
relationship between density and the diversity of species and seed size.
Several more diverse communities exhibited characteristics of colonizing
species, such as lower wood density and smaller seeds. On Barro Colorado
Island, Panama, Muller-Landau (2004) also found a weak negative correlation
between the wood density and rate of adult mortality and the rate of relative
growth of trees and saplings. In other words, short-lived species with higher
rates of growth have lower wood density. Similar results are reported by
Favrichon (1994) and Suzuki (1999).
In open forests in the state of Acre, in southwestern
Amazonia, low wood density is believed to result from both phytogeographical
and ecological factors (França, 2002). For example, trees in the family Bombacaceae,
which are typically light weight, are more abundant in all forest types of this
region. A larger number of pioneer tree species and of fast-growing species may
also be responsible for the low mean density of disks taken at breast height
from trees in a bamboo-dominated forest of this region: only 0.51 g.cm-3
determined at 80oC. Common pioneer taxa here include Acacia
polyphylla, Apeiba sp., Jacaratia sp., Cavanillesia
hylogeiton, Ceiba sp. and Cecropia sciadophylla (Oliveira,
2000). In these environments, fast-growing species are favored by the
occurrence of fertile soils (Cambisols or Inceptisols), by extensive temporary
gaps resulting from natural disturbance by bamboo (Guadua sp.) and from
the periodic and synchronized death of this bamboo. Schnitzer et al. (2000), in
a study of 428 treefall gaps in tropical forest on Barro Colorado Island,
Panama, found a similar correlation between liana abundance and the abundance
of pioneer trees.
4.2.
Methodological uncertainties in density determination
An important source of uncertainty
in the available density data for Amazonia is species identification in forest
inventories. Fearnside (1997a) found that many published inventories are based
on common names. When the scientific names are reported, they are not based on
formal botanical identification, but rather use tables equating common and
scientific designations. According to Pires (1978), more than 90% of the
identifications used in the inventories conducted by the Food and Agriculture
Organization of the United Nations (FAO) in Amazonia could be in error at the
species level because they have been based on common names. The data in the FAO
inventory (Heinsdijk, 1958) have been used for calculations of biomass and
emissions of carbon in Amazonia because they are representative of several
vegetation types (Brown et al., 1989). These uncertainties demonstrate the
importance of studies to determine density with correct identification of the
species.
In the present study, a test was
conducted on the reliability of the common names supplied by a local woodsman (mateiro).
These were transformed to scientific names using three guides: Catalog of Trees
of Brazil (Camargos et al., 2001), Flora of the Reserva Ducke (Ribeiro et al.,
1999) and Common Names of Amazonian Plants (Silva et al., 1977), Appendix 2.
All of the trees also had botanical specimens identified in the herbarium, so
the correct scientific names were also known. Only 53% of the scientific names
inferred from the common names supplied by the mateiro proved to be
correct. The common names and scientific names were considered to be equivalent
when the common name mentioned by the mateiro was similar to one of the
common names mentioned in the literature, or to one of the names listed when
the common name is a compound word. Mistakes sometimes occurred when the mateiro
attributed different names for a given species, or when common names were
identified in different places. The mateiro was sometimes unable to
identify the same species that he had identified previously.
Another source of uncertainty is the
use of different methods for obtaining density. The following types have been
reported (1) apparent densities, with a moisture content of 12% (g water/100 g
oven-dry weight), based on the methodology of COPANT (1973); (2) green density,
such as the data on 50 species published by IBAMA (Souza et al., 2002), or for
40 species occurring in the Tapajós National Forest (Fedalto, 1989), and (3)
density based on the volume re-hydrated from green wood samples, such as 75
species collected in the Curuá-Una forest management research area in Pará
(Brazil, IBDF, 1988; Vol. 2), 23 species sampled in forests in the state of
Amapá (Brazil, INPA/CPPF, 1993) and 40 species in the area of the Balbina
hydroelectric dam (Brazil, INPA/CPPF, 1991). This has hindered the obtaining of
consistent values for basic density using the ratio of dry weight to true green
volume in the living tree. In some references, the green volume refers to wood
that has been allowed to air dry and is later re-hydrated until saturation, or
that has been sampled green and later saturated (Brazil, IBDF, 1988; Vol. 2, p.
29).
Time for complete drying of the
samples was highly variable; some required more than 20 days at a temperature
of 103 ± 2 ºC to achieve a stable weight. Thus basic density will be
overestimated if drying times are limited to a few days and standardized for
different species and sample sizes. The density obtained from drying at 80ºC
was significantly higher than the density obtained at 103ºC (Table 1). Since
the weight obtained at 80oC was considered dry after stabilization (constant
weight for three consecutive measurements), the loss of additional weight when
dried at 103oC
could represent water that is chemically bound to the cell wall, as well as
organic compounds that are volatilized at the higher temperature.
Presence of hollows means that
central heartwood is lost from the disk, causing a bias toward more external wood,
which was usually less dense in this study. Hollows were found in 10% of the
trees, including 7% at breast height.
But hollows accounted for just 0.7% of the total stand bole volume after
adjusting for size-class frequencies typical of a large inventory. Our method,
in which density is based on a cross-sectional disc instead of small solid wood
samples, avoids bias of the density results from the presence of hollows.
4.3.
Wood density and biomass estimates
Studies of wood density for
species in Amazonia are important for biomass estimates because this
information is necessary for conversion of volume data from forest inventories
to biomass (Houghton et al., 2001; Brown, 1997; Brown and Lugo, 1992):
TAGB = Inventoried
volume * VEF * WD * BEF (eq.
3)
Where:
TAGB
= Total above-ground biomass of standing trees (≥10 cm DBH; Mg ha-1)
Inventoried
volume = commercial volume of the boles above the minimum DBH inventoried (m3 ha-1).
Usually, minimum inventoried DBH is between 25 and 30 cm,
VEF =
Volume Expansion factor, to represent the volume of boles between 10 cm and the
minimum DBH inventoried,
WD =
Wood density, stand average of all boles,
BEF
= Biomass Expansion Factor (expands bole biomass to all above-ground biomass,
for all trees ≥ 10 cm DBH).
The
following values are assumed for Amazonia, in accord with Houghton et al.
(2001, citing Brown and Lugo, 1992):
VEF = 1.25 for dense forests, or 1.5 for other Amazonian forests;
WD = 0.69,
BEF = exp {3.213–0.506 Ln SB}, for SB < 190 Mg ha-1;
BEF = 1.74, for SB > 190 Mg ha-1;
SB = Stand biomass (biomass of the boles) ≥10 cm DBH = inventoried
volume * VEF * WD
For
estimates based on volumetric data, wood density of 0.69 g.cm-3 has
been used as mean value for Brazilian Amazonia (Houghton et al., 2001;
Fearnside, 1997a; Brown, 1997; Brown and Lugo, 1992; Brown et al., 1989). This
value may be subject to the overestimation biases reported in this paper.
For
dense forest of Central Amazonia, the density difference between heartwood and whole
disk will lead to an overestimate of 5.3%, the difference between the whole
disk at breast height and the average total bole density is 4.3%, while the
effect of re-hydrating oven-dried heartwood is an overestimate of 2.5%. So if
average bole density of a stand is based on re-hydrating oven-dried samples of
heartwood taken near the center of a disk at breast height, there will be a
~12.1% overestimate of both density and biomass (eq. 3). A fourth tendency for
overestimation is the exclusion of bark from most samples used in prior
studies. A fifth tendency in the same direction will result from using standard
drying times. Re-hydration times of 14 days or less are a sixth source of
overestimate, as shown in this study. Re-hydration of samples larger than the
small (12 cm3 ) blocks used in this study will mean even greater
overestimates than those reported here, but re-hydration of wood more porous
than the heartwood used here or re-hydrating air-dried samples, will reduce or
eliminate the bias.
To
what extent do previously published lists of wood density by species include
all these biases toward overestimate? In the case of data on wood density of
Amazonian trees published by the Coordination of Research on Forest Products of
the National Institute for Research in Amazonia (INPA), the Laboratory of
Forestry Research of the Brazilian Institute for the Environment and Renewable
Natural Resources (IBAMA) and the Center for Wood Technology of the
Superintendency for Development of Amazonia (SUDAM), samples were taken at
random from different sections of the bole, based on the norms of COPANT
(Brazil, IBDF, 1981,1988; Brazil, INPA/CPPF, 1991, pp. 5 and 7; Brazil,
INPA/CPPF, 1993, p. 8; Brazil, IBAMA, 1997). Sampling protocols for basic
density followed by the Brazilian Institute for Forest Development (IBDF, 1981,
1988) are random with respect to height along the bole, but the center of each
specimen was, on average, just 5.3 cm from center of the disk. Re-hydration
protocols used by IBDF (1981, 1988) called for immersing green wood “for a long
period” then drying at 103oC, and so will be more efficient at
regaining fresh volume than re-hydration that begins from dried samples.
The
net effect of biases in the estimate of stand density and biomass using
published wood density data can be better examined by comparing the results of
this study with three previous estimates for the Central Amazon, which matched
forest inventories to published lists of wood density by taxon. In those
studies, overestimates of average density of the
entire bole -- here presumed to be 0.67 g.cm-3 -- were 6%, 4% and 0%
(Fearnside, 1997a; Baker et al., 2004; Muller-Landau, 2004). Based on
samples collected without bark and at breast height, plus published data at the
species level, Muller-Landau (2004) found an inventory-adjusted average basic
density of 0.71 for 112 trees from Central Amazon dense forest. Fearnside
(1997a) reported an average density of 0.70 g.cm-3 for this same region, using
published density data and inventories. His number is identical to
the value found in the present study at breast height, but is higher than the 0.67 g.cm-3 mean basic density of the entire
bole with bark. Baker et al. (2004) found 0.67 g.cm-3 to be the
stand level average for wood density in the Central Amazon based on 11 ha of
dense forest inventory and lists of wood density covering 584 species of
Amazonian trees. Their matches were made mostly at the genus or family level,
i.e. were matched to related species or related genera. This may introduce a
bias toward the more workable (less dense) commercial timbers and toward trees
harvested on more fertile soil than the Central Amazon. In the case of Baker et
al. (2004), these two biases toward lower wood density appear to have fully
compensated the density overestimation biases reported in this paper.
5. Significance for global change estimates
The adjustments to wood density values used for
calculating the biomass of Amazonian forests and the greenhouse gas emissions
that result when these forests are cleared have important implications for
global change. For example, an estimate of net committed emissions of 249 × 106
Mg CO2-equivalent C/year for Brazilian Amazonia in 1990 (midpoint of
high- and low-trace gas scenarios, including effects of CO2, CH4
and N2O), of which 237 × 106 Mg CO2-equivalent
C/year was from net removal of biomass (updated from Fearnside, 2000a), would
be reduced by 14 × 106 Mg CO2-equivalent C/year, or 5.7%.
The percentage reduction in net emissions is greater than the 5.3% reduction in
gross emissions because the estimates for biomass accumulation in regenerating
secondary forest are unaffected by the wood density adjustments.
Decreases of similar proportion would apply throughout
the tropics. An annual gross emission of 2.0 × 109 Mg of carbon
(without considering trace-gas effects) from biomass in the tropics during the
1980s (Fearnside, 2000b, p. 128) would be reduced by 113 × 106 Mg C
annually, assuming the same adjustment applies to all tropical forests.
This
adjustment would be increased to approximately 132 Mg
CO2-equivalent
C/year if the
effect of trace gases is considered (15.5% ± 9.5%, based on Fearnside, 1997b).
6.
Conclusions
Wood density estimates
that have been widely used as the basis of estimating Amazon forest biomass
need to be adjusted downward by 5.3% for density variation in the
cross-sectional disk. Some studies will
require an additional 4.3% downward adjustment for density variation along the
length of the bole.
The present study’s
results for wood density imply a 5.3% downward adjustment for estimates of
primary forest biomass in Amazonia, and adjustment by the same amount of
estimates of gross emissions of greenhouse gases from deforestation. Because
regrowth estimates are unaffected by the adjustments, net committed emissions
would be lowered by a slightly greater percentage: 5.7% in the case of
Amazonian deforestation. However, these adjustments do not resolve differences
among the various estimates that exist for biomass and emissions, since all
estimates have been based on nearly identical assumptions regarding wood
density in tropical forests.
Acknowledgements
The National Council of Scientific and Technological
Development (CNPq AI 470765/01-1) and the National Institute for Research in
the Amazon (INPA PPI 1-3620) provided financial support. Two anonymous reviewers made valuable
comments.
References
Brazil, INMET,
2003. Instituto Nacional de Meteorologia. www.inmet.gov.br. Accessed 12 August
2003.
Brazil,
INPA/CPPF, 1991. Catálogo de madeiras da Amazônia. Coordenação de Pesquisa de
Produtos Florestais. Instituto Nacional de Pesquisas da Amazônia. Manaus,
Amazonas, Brazil. 153 pp.
Brazil,
INPA/CPPF, 1993. Catálogo de madeiras do Amapá: características tecnológicas.
Coordenação de Pesquisas em Produtos Florestais. Instituto Nacional de
Pesquisas da Amazônia, Manaus, Amazonas, Brazil. 58 pp.
Brazil, Projeto RADAMBRASIL,
1978. Folha SA.20, Levantamento de Recursos Naturais, Manaus. Departamento
Nacional de Produção Mineral, Rio de Janeiro, Brazil. Vol. 18. 747
pp.
Brown, S., Lugo, A.
E., 1992. Aboveground biomass estimates for tropical moist forests of the
Brazilian Amazon. Interciencia 17, 8-18.
Brown, S., Gillespie, A. J. R., Lugo, A. E.,
1989. Biomass estimation methods for tropical forest with
applications to forest inventory data. For. Sci. 35, 881-902.
Camargos, J. A. A.,
Coradin, V. T. R., Czarneski, C. M., Oliveira, D. de., Meguerditchian, I.,
2001. Catálogo de árvores do Brasil. Instituto Brasileiro do Meio Ambiente e
dos Recursos Naturais Renováveis. Laboratório de Produtos Florestais. Edições
IBAMA, Brasília, DF, Brazil. 896 pp.
Chambers, J. Q.,
Santos, J., Ribeiro, R. J., Higuchi, N., 2001. Tree damage.
allometric relationships, and above-ground net primary production in central Amazon Forest. Forest Ecol. and
Manage. 152, 73-84.
Chimelo, J. P.,
1992. Relacionamento da anatomia da madeira com suas propriedades físicas e
mecânicas. Escola Superior de Agricultura “Luis de Queiroz”, . Escola Superior
de Agricultura “Luis de Queirroz”, Universidade de São Paulo (ESALQ/USP),
Piracicaba, São Paulo, Brazil (mimeographed). 25 pp.
DeZeeuw,
C., 1965. Variability in wood. In: Côté, W.A. (Ed.), Cellular ultrastructure of
woody plants. Syracuse University
Press. Syracuse, New
York, U.S.A. pp.
457-471.
Favrichon, V., 1994.
Classification des espèces arborées en groupes fontionnels en vue de la
réalisation d'un modèle de dynamique de peuplement en forêt Guyannaise. Revue d'Ecologie Terre et Vie
49, 379-402.
Fearnside, P.M., 1997b. Greenhouse gases from deforestation in
Brazilian Amazonia: Net committed emissions. Climatic Change 35, 321-360.
Fearnside,
P.M., 2000a. Greenhouse gas emissions
from land-use change in Brazil's Amazon region. In: Lal, R., Kimble, J.M., Stewart, B.A. (Eds.), Global Climate Change and
Tropical Ecosystems. Advances in Soil Science. CRC Press, Boca Raton, Florida, U.S.A. pp. 231-249
Fearnside,
P.M., 2000b. Global warming and tropical
land-use change: Greenhouse gas emissions from biomass burning, decomposition
and soils in forest conversion, shifting cultivation and secondary vegetation. Climatic Change 46, 115-158.
Fedalto, L. C.,
Mendes, I. C. A., Coradin, V. T. R., 1989. Madeiras da Amazônia: descrição do
lenho de 40 espécies ocorrentes na Floresta Nacional do Tapajós. Instituto
Brasileiro do Meio Ambiente e dos Recursos Naturais Renováveis (IBAMA),
Brasília, DF, Brazil. 156 pp.
Hacke, U.G.,
Sperry, J.S., Pockman, W.T., Davis, S.D., McCulloh, K.A., 2001. Trends
in wood density and structure are linked to prevention of xylem implosion by
negative pressure. Oecologia 126, 457-461.
Ilic,
J., Boland, D., McDonald, M., Downes, G., Blakemore, P., 2000. Woody Density
Phase 1 - State of Knowledge.
National Carbon Accounting System Technical Report 18, Australian Greenhouse
Office, Canberra, Australia.
228 pp.
Kollmann,
F. F. P., Côté, W.A. Jr., 1968. Principles of wood science and technology solid
wood., v. 1. Spring-Verlag, New
York, U.S.A. 592
pp.
Magnago,
H., Barreto, R.A.A., Pastore, U., 1978. Projeto RADAMBRASIL. Folha
SA.20. Parte IV-Vegetação, Departamento Nacional de Produção Mineral, Rio de
Janeiro, Brazil. pp. 413-530.
Melo, J.E.,
Coradin, V.T.R., Mendes, J.C., 1990. Classes de densidade de madeira para a
Amazônia Brasileira. In: Anais do Congresso Florestal Brasileiro, 6. Campos do
Jordão, São Paulo, Sociedade Brasileira de Silvicultura, São Paulo, SP, Brazil.
Vol. 3. pp. 695-699.
Muller-Landau,
H.C. (2004) Interspecific and inter-site variation in wood specific gravity of
tropical trees. Biotropica 36(1): 20-32.
Nelson, B.W., Mesquita, R.C.G., Pereira,
J.L.G., Souza, S.G.A., Batista, G.T., Couto, L.B., 1999. Allometric regressions
for improved estimate of secondary forest biomass in the central Amazon. Forest
Ecol. and Manage. 117, 149-167.
Nogueira, E.M., Nelson, B.W., 2003. Ocorrência de
árvores ocadas em floresta densa na Amazônia Central. 54º Congresso Nacional de
Botânica, Belém - Ananindeua – Pará. Sociedade Brasileira de Botânica, São
Paulo, SP, Brazil. (Abstract). CD-ROM.
Oliveira, A.C.A., 2000. Efeitos do Bambu Guadua weberbaueri
Pilger sobre a Fisionomia e Estrutura de uma Floresta no Sudoeste da Amazônia.
Masters thesis in Ecology, Instituto Nacional de Pesquisas da Amazônia-Fundação
Universidade Federal do Amazonas, Manaus, Amazonas, Brazil . 103 pp.
Pinto, A. C. M., Higuchi,
N., Iida, S., Santos, J. Dos, Ribeiro, R. J., Rocha, R. M., Silva, R. P. da.,
2003. Padrão de distribuição espacial de espécies florestais que ocorrem na
região de Manaus-AM. In: Higuchi, N., Santos, J. dos., Sampaio, P.T.B.,
Marenco, R. A., Ferraz, J., Sales, P. C. de., Saito, M., Matsumoto, S. (Eds.).
Projeto Jacarandá, Fase II: Pesquisas florestais na Amazônia Central.
CPST/INPA, Manaus Amazonas, Brazil. 252 pp.
Pires,
J. M., 1978. The forest ecosystems of the Brazilian Amazon: Description.
functioning and research needs. In: United Nations Educational. Scientific and
Cultural Organization (UNESCO)/United Nations Environmental Programme (UNEP)/Food
and Agriculture Organization of the United Nations (FAO). Tropical Forest
Ecosystems: A State of Knowledge
Report, UNESCO, Paris, France.
pp. 607-627.
Prance, G.T., Rodrigues,
W.A., da Silva, M.F., 1976. Inventário florestal de um hectare de mata de
terra-firme, Km 30 da Estrada Manaus-Itacoatiara. Acta
Amazonica 6, 9-35.
Putz, F.E., Coley,
P.D., Lu. K., Montalvo, A., Aiello, A., 1983. Uprooting and snapping of trees:
structural determinants and ecological consequences. Canad. J. For. Res. 13,
1011-1020.
Ribeiro, J.E.L. da S.,
Hopkins, M.J.C., Vicentini, A., Sothers, C.A., Costa, M.A. da S., Brito, J.M.
de., Souza, M.A.D. de., Martins, L.H.P., Lohmann, L.G., Assunção, P.A. C.L.,
Pereira, E. da C., Silva, C.F. da., Mesquita, M.R., Procópio, L.C., 1999. Flora da Reserva
Ducke: guia de identificação das plantas vasculares de uma floresta de
terra-firme na Amazônia Central. INPA/DfID, Manaus, Amazonas, Brazil. 816 pp.
Ribeiro, R.J., 1996. Estudos
de função de forma para espécies florestais de terra-firme da Amazônia.Masters
thesis in forest sciences, Instituto Nacional de Pesquisas da Amazônia/Fundação
Universidade do Amazonas, Manaus, Amazonas, Brazil. 76 pp.
Santos, J., 1996. Análise de Modelos de Regressão para Estimar a
Fitomassa da Floresta Tropical Úmida de Terra-firme da Amazônia Brasileira. Doctoral
dissertation in forest sciences, Universidade Federal de Viçosa, Viçosa, Minas
Gerais, Brazil. 121 pp.
Silva, J.C.,
1984. Parâmetros da densidade na qualidade da madeira. . Escola Superior de
Agricultura “Luis de Queirroz”, Universidade de São Paulo (ESALQ/USP),
Piracicaba, São Paulo, Brazil. 82 pp.
Silva, M.F. da.,
1977. Nomes vulgares de plantas amazônicas. Instituto Nacional de Pesquisas da
Amazônia (INPA), Manaus, Amazonas, Brazil. 222 pp.
Simpson,
W., TenWolde, A., 1999. Physical properties and moisture relations of wood.
Chapter. 3. In: Forest
Products Laboratory. Wood Handbook-wood as an engineering material. Gen. Tech.
Rep. FPL-GTR-113. U.S.
Department of Agriculture, Forest
Service. Forest
Products Laboratory, Madison, Wisconsin, U.S.A. 463
pp.
Souza,
M.H. de., Magliano, M.M., Camargos, J.A.A., Souza, M.R. de., 2002. Madeiras
tropicais brasileiras/Brazilian tropical woods. Instituto Brasileiro do Meio
Ambiente e dos Recursos Naturais Renováveis, 2. ed. edições IBAMA, Brasília,
DF, Brazil. 152 pp.
Suzuki,
E., 1999. Diversity in specific gravity and water content of wood among Bornean
tropical rainforest trees. Ecol. Res. 14, 211-224.
Ter
Steege, H., Hammond,
D.S., 1996. Forest
management in the Guianas: Ecological and evolutionary constraints on timber production.
BOS NiEuwsletter 15, 62-69.
Ter
Steege, H., Hammond,
D.S., 2001. Character convergence, diversity, and disturbance in tropical rain
forest in Guyana. Ecology 82,
3197-3212.
Trugilho, P.F.,
Silva, D.A., Frazão, F.J.L., Matos, J.L.M., 1990. Comparação de métodos de
determinação de densidade básica em madeira. Acta Amazonica 20, 307-319.
Vital, B.R., 1984. Métodos de determinação da densidade da madeira.
Boletim Técnico. 2, Sociedade de Investigações Florestais (SIF), Viçosa, Minas
Gerais, Brazil. 21 pp.
Yamazaki, D.R.,
Costa, A.M.R., Azevedo, W.P., 1978. Projeto RADAMBRASIL, Folha SA.20, Parte
III-Pedologia. Departamento Nacional de Produção Mineral, Rio de Janeiro, RJ,
Brazil. pp. 247-410.
Wiemann,
M.C., Williamson, G.B., 1988. Extreme radial changes in wood specific gravity
in some tropical pioneers. Wood and Fiber Science 20, 344-349.
Wiemann,
M.C., Williamson, G.B., 1989. Radial gradients in the specific gravity of wood
in some tropical and temperate trees. Forest
Science 35, 197-210.
Wiemann,
M.C., Williamson, G.B., 2002. Geographic variation in wood specific gravity:
Effects of latitude. temperature and precipitation. Wood and Fiber Science 34,
96-107.
Woodcock,
D.W., 2000. Wood specific gravity of trees and forest types in the southern
Peruvian Amazon. Acta Amazonica 30, 589-599.